| Test Name | No. of Questions | Marks (of each) | Time | Take Test |
|---|---|---|---|---|
| Test-1 | 12 | 3 | 40 Minutes | |
| Test-2 | 12 | 3 | 40 Minutes | |
| Test-3 | 12 | 3 | 40 Minutes | |
| Test-4 | 12 | 3 | 40 Minutes | |
| Test-5 | 12 | 3 | 40 Minutes | |
| Test-6 | 12 | 3 | 40 Minutes | |
| Test-7 | 12 | 3 | 40 Minutes | |
| Test-8 | 12 | 3 | 40 Minutes |
Instructions
Each of these items has a question followed by two statements. As the answer,
Type 1, If the question can be answered with the help of statement I alone,
Type 2, If the question can be answered with the help of statement II alone,
Type 3, If both the statement I and statement II are needed to answer the question, and
Type 4, If the question cannot be answered even with the help of both the statements.
A train started from Station A, developed engine trouble and reached Station B, 40 minutes late. What is the distance between Stations A and B?
- The engine trouble developed after travelling 40 km from Station A and the speed reduced to 1/4th of the original speed.
- The engine trouble developed after travelling 40 km from station A in two hours and the speed reduced to ¼th of the original speed.
Each of these items has a question followed by two statements. As the answer,
Type 1, If the question can be answered with the help of statement I alone,
Type 2, If the question can be answered with the help of statement II alone,
Type 3, If both the statement I and statement II are needed to answer the question, and
Type 4, If the question cannot be answered even with the help of both the statements.
A train started from Station A, developed engine trouble and reached Station B, 40 minutes late. What is the distance between Stations A and B?
- The engine trouble developed after travelling 40 km from Station A and the speed reduced to 1/4th of the original speed.
- The engine trouble developed after travelling 40 km from station A in two hours and the speed reduced to ¼th of the original speed.
Question 1.
A
1
B
2
C
3
D
4
Question 2.
Every day Neera’s husband meets her at the city railway station at 6.00 p.m. and drives her to their residence. One day she left early from the office and reached the railway station at 5.00 p.m. She started walking towards her home, met her husband coming from their residence on the way and they reached home 10 minutes earlier than the usual time. For how long did she walk?
A
1 hour
B
50 minutes
C
1/2 hour
D
55 minutes
Question 3.
A ship leave on a long voyage. When it is 18 miles from the shore, a seaplane, whose speed is ten times that of the ship, is sent to deliver mail. How far from the shore does the seaplane catch up with the ship?
A
24 miles
B
25 miles
C
22 miles
D
20 miles
Instructions
Q started to move from point B towards point A exactly an hour after P started from A in the opposite direction. Q’s speed was twice that of P. When P had covered one-sixth of the distance between the points A and B, Q had also covered the same distance.
Q started to move from point B towards point A exactly an hour after P started from A in the opposite direction. Q’s speed was twice that of P. When P had covered one-sixth of the distance between the points A and B, Q had also covered the same distance.
Question 4.
How many more hours would P (compared to Q) take to complete his journey?
A
4
B
5
C
6
D
7
Instructions
Q started to move from point B towards point A exactly an hour after P started from A in the opposite direction. Q’s speed was twice that of P. When P had covered one-sixth of the distance between the points A and B, Q had also covered the same distance.
Q started to move from point B towards point A exactly an hour after P started from A in the opposite direction. Q’s speed was twice that of P. When P had covered one-sixth of the distance between the points A and B, Q had also covered the same distance.
Question 5.
How many hours would P take to reach B?
A
2
B
5
C
6
D
12
Instructions
Q started to move from point B towards point A exactly an hour after P started from A in the opposite direction. Q’s speed was twice that of P. When P had covered one-sixth of the distance between the points A and B, Q had also covered the same distance.
Q started to move from point B towards point A exactly an hour after P started from A in the opposite direction. Q’s speed was twice that of P. When P had covered one-sixth of the distance between the points A and B, Q had also covered the same distance.
Question 6.
The point where P and Q would meet is
A
Closer to A
B
Exactly between A and B
C
Closer to B
D
P and Q will not meet at all
Question 7.
The winning relay team in a high school sports competition clocked 48 minutes for a distance of 13.2 km. Its runners A, B, C and D maintained speeds of 15 kmph, 16 kmph, 17 kmph, and 18 kmph respectively. What is the ratio of the time taken by B to than taken by D?
A
5 : 16
B
5:17
C
9:8
D
8:9
Question 8.
A and B walk from X to Y, a distance of 27 km at 5 kmph and 7 kmph respectively. B reaches Y and immediately turns back meeting A at Z. What is the distance from X to Z?
A
25 km
B
22.5 km
C
24 km
D
20 km
Question 9.
Shyam went from Delhi to Shimla via Chandigarh by car. The distance from Delhi to Chandigarh is times the distance from Chandigarh to Shimla. The average speed from Delhi to Chandigarh was half as much again as that from Chandigarh to Shimla. If the average speed for the entire journey was 49 kmph. What was the average speed from Chandigarh to Shimla?
A
39.2 kmph
B
63 kmph
C
42 kmph
D
None of these
Question 10.
Two towns A and B are 100 km apart. A school is to be built for 100 students of town B and 30 students of Town A. Expenditure on transport is Rs. 1.20 per km per student. If the total expenditure on transport by all 130 students is to be as small as possible, then the school should be built at
A
33 km from Town A.
B
33 km from Town B
C
Town A
D
Town B
Question 11.
A man can walk up a moving ‘up’ escalator in 30 s. The same man can walk down this moving ‘up’ escalator in 90 s. Assume that his walking speed is same upwards and downwards. How much time will he take to walk up the escalator, when it is not moving?
A
30 s
B
45 s
C
60 s
D
90 s
Question 12.
I live X floors above the ground floor of a high-rise building. It takes me 30 s per floor to walk down the steps and 2 s per floor to ride the lift. What is X, if the time taken to walk down the steps to the ground floor is the same as to wait for the lift for 7 min and then ride down?
A
4
B
7
C
14
D
15
Question 13.
In a race of 200 m run, A beats S by 20 m and N by 40 m. If S and N are running a race of 100 m with exactly same speed as before, then by how many metres will S beat N?
A
11.11 m
B
10 m
C
12 m
D
25 m
Question 14.
A man travels from A to B at a speed x km/hr. He then rests at B for x hours. He then travels from B to C at a speed 2x km/hr and rests for 2x hours. He moves further to D at a speed twice as that between B and C. He thus reaches D in 16 hr. If distances A-B, B-C and C-D are all equal to 12 km, the time for which he rested at B could be
A
3 hr
B
6 hr
C
2 hr
D
4 hr
Question 15.
A man travels three-fifths of a distance AB at a speed 3a, and the remaining at a speed 2b. If he goes from B to A and return at a speed 5c in the same time, then
A
+ =
B
a + b = 99
C
+ =
D
None of these
Question 16.
In a mile race, Akshay can be given a start of 128 m by Bhairav. If Bhairav can give Chinmay a start of 4 m in a 100 m dash, then who out of Akshay and Chinmay will win a race of one and half miles, and what will be the final lead given by the winner to the loser? (One mile is 1,600 m.)
A
Akshay, mile
B
Chinmay, mile
C
Akshay, mile
D
Chinmay, mile
Instructions
Direction: Answer the question based on the following information.
In a locality, there are five small cities: A, B, C, D and E. The distances of these cities from each other are as follows.
AB = 2 km AC = 2km AD > 2 km AE > 3 km BC = 2 km
BD = 4 km BE = 3 km CD = 2 km CE = 3 km DE > 3 km
Direction: Answer the question based on the following information.
In a locality, there are five small cities: A, B, C, D and E. The distances of these cities from each other are as follows.
AB = 2 km AC = 2km AD > 2 km AE > 3 km BC = 2 km
BD = 4 km BE = 3 km CD = 2 km CE = 3 km DE > 3 km
Question 17.
If a ration shop is to be set up within 3 km of each city, how many ration shops will be required?
A
1
B
2
C
3
D
4
Instructions
Direction: Answer the question based on the following information.
In a locality, there are five small cities: A, B, C, D and E. The distances of these cities from each other are as follows.
AB = 2 km AC = 2km AD > 2 km AE > 3 km BC = 2 km
BD = 4 km BE = 3 km CD = 2 km CE = 3 km DE > 3 km
Direction: Answer the question based on the following information.
In a locality, there are five small cities: A, B, C, D and E. The distances of these cities from each other are as follows.
AB = 2 km AC = 2km AD > 2 km AE > 3 km BC = 2 km
BD = 4 km BE = 3 km CD = 2 km CE = 3 km DE > 3 km
Question 18.
If a ration shop is to be set up within 2 km of each city, how many ration shops will be required?
A
2
B
3
C
4
D
5
Instructions
Answer the next 2 questions based on the following information.
A thief, after committing the burglary, started fleeing at 12 noon, at a speed of 60 km/hr. He was then chased by a policeman X. X started the chase, 15 min after the thief had started, at a speed of 65 km/hr.
Answer the next 2 questions based on the following information.
A thief, after committing the burglary, started fleeing at 12 noon, at a speed of 60 km/hr. He was then chased by a policeman X. X started the chase, 15 min after the thief had started, at a speed of 65 km/hr.
Question 19.
If another policeman had started the same chase along with X, but at a speed of 60 km/hr, then how far behind was he when X caught the thief?
A
18.75 km
B
15 km
C
21 km
D
37.5 km
Question 20.
At what time did X catch the thief?
A
3.30 p.m.
B
3 p.m.
C
3.15 p.m.
D
None of these
Question 21.
What is X's average speed for the entire journey (to and fro)?
A
176 kmph
B
180 kmph
C
165 kmph
D
Data insufficient
Instructions
Direction: Answer the question based on the following information.
Boston is 4 hr ahead of Frankfurt and 2 hr behind India. X leaves Frankfurt at 6 p.m. on Friday and reaches Boston the next day. After waiting there for 2 hr, he leaves exactly at noon and reaches India at 1 a.m. On his return journey, he takes the same route as before, but halts at Boston for 1hr less than his previous halt there. He then proceeds to Frankfurt.
Direction: Answer the question based on the following information.
Boston is 4 hr ahead of Frankfurt and 2 hr behind India. X leaves Frankfurt at 6 p.m. on Friday and reaches Boston the next day. After waiting there for 2 hr, he leaves exactly at noon and reaches India at 1 a.m. On his return journey, he takes the same route as before, but halts at Boston for 1hr less than his previous halt there. He then proceeds to Frankfurt.
Question 22.
If X had started the return journey from India at 2.55 a.m. on the same day that he reached there, after how much time would he reach Frankfurt?
A
24 hr
B
25 hr
C
26 hr
D
Data insufficient
Instructions
Direction: Answer the question based on the following information.
Boston is 4 hr ahead of Frankfurt and 2 hr behind India. X leaves Frankfurt at 6 p.m. on Friday and reaches Boston the next day. After waiting there for 2 hr, he leaves exactly at noon and reaches India at 1 a.m. On his return journey, he takes the same route as before, but halts at Boston for 1hr less than his previous halt there. He then proceeds to Frankfurt.
Direction: Answer the question based on the following information.
Boston is 4 hr ahead of Frankfurt and 2 hr behind India. X leaves Frankfurt at 6 p.m. on Friday and reaches Boston the next day. After waiting there for 2 hr, he leaves exactly at noon and reaches India at 1 a.m. On his return journey, he takes the same route as before, but halts at Boston for 1hr less than his previous halt there. He then proceeds to Frankfurt.
Question 23.
If his journey, including stoppage, is covered at an average speed of 180 mph, what is the distance between Frankfurt and India?
A
3,600 miles
B
4,500 miles
C
5,580 miles
D
Data insufficient
Question 24.
An express train travelling at 80 km/hr overtakes a goods train, twice as long and going at 40 km/hr on a parallel track, in 54 s. How long will the express train take to cross a platform of 400 m long?
A
36 s
B
45 s
C
27 s
D
None of these
Instructions
Answer the next 3 questions based on the following information.
A certain race is made up of three stretches: A, B and C, each 2 km long, and to be covered by a certain mode of transport. The following table gives these modes of transport for the stretches, and the minimum and maximum possible speeds (in km/hr) over these stretches. The speed over a particular stretch is assumed to be constant. The previous record for the race is 10 min.
Min
Max
A
Car
40
60
B
Motorcycle
30
50
C
Bicycle
10
20
Answer the next 3 questions based on the following information.
A certain race is made up of three stretches: A, B and C, each 2 km long, and to be covered by a certain mode of transport. The following table gives these modes of transport for the stretches, and the minimum and maximum possible speeds (in km/hr) over these stretches. The speed over a particular stretch is assumed to be constant. The previous record for the race is 10 min.
| Min | Max | ||
| A | Car | 40 | 60 |
| B | Motorcycle | 30 | 50 |
| C | Bicycle | 10 | 20 |
Question 25.
Mr Tortoise completes the race at an average speed of 20 km/hr. His average speed for the first two stretches is four times that for the last stretch. Find the speed over stretch C.
A
15 km/hr
B
12 km/hr
C
10 km/hr
D
This is not possible
Question 26.
Mr Hare completes the first stretch at the minimum speed and takes the same time for stretch B. He takes 50% more time than the previous record to complete the race. What is Mr Hare's speed for the stretch C?
A
10.9 km/hr
B
13.3 km/hr
C
17.1 km/hr
D
None of these
Question 27.
Anshuman travels at minimum speed by car over A and completes stretch B at the fastest speed. At what speed should he cover stretch C in order to break the previous record?
A
Maximum speed for C
B
Minimum speed for C
C
This is not possible
D
None of these
Question 28.
I started climbing up the hill at 6 a.m. and reached the top of the temple at 6 p.m. Next day I started coming down at 6 a.m. and reached the foothill at 6 p.m. I walked on the same road. The road is so short that only one person can walk on it. Although I varied my pace on my way, I never stopped on my way. Then which of the following must be true?
A
My average speed downhill was greater than that of uphill
B
At noon, I was at the same spot on both the days.
C
There must be a point where I reached at the same time on both the days.
D
There cannot be a spot where I reached at the same time on both the days.
Question 29.
Distance between A and B is 72 km. Two men started walking from A and B at the same time towards each other. The person who started from A travelled uniformly with average speed of 4 km/hr. While the other man travelled with varying speed as follows: in the first hour his speed was 2 km/hr, in the second hour it was 2.5 km/hr, in the third hour it was 3 km/hr, and so on. When will they meet each other?
A
7 hr
B
10 hr
C
35 km from A
D
Mid-way between A and B
Instructions
Directions: Answer the questions based on the following information.
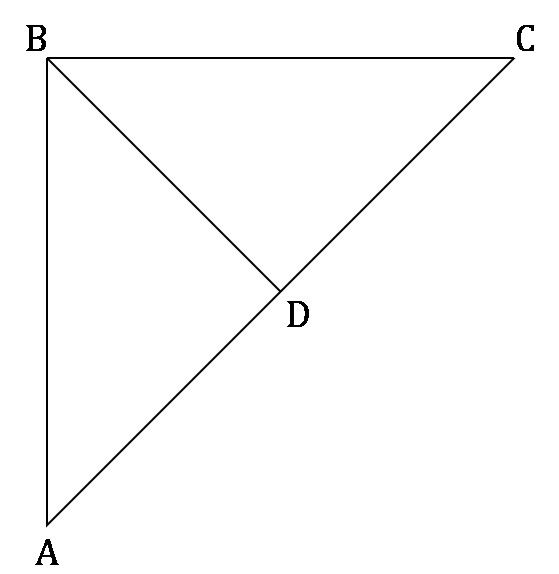
A road network (shown in the figure below) connects cities A, B, C and D. All road segments are straight lines. D is the mid-point on the road connecting A and C. Roads AB and BC are at right angles to each other with BC shorter than AB. The segment AB is 100 km long.
Ms X and Mr Y leave A at 8.00 a.m., take different routes to city C and reach at the same time. X takes the highway from A to B to C and travels at an average speed of 61.875 kmph. Y takes the direct route AC and travels at 45 kmph on segment AD. Y’s speed on segment DC is 55 kmph.
Directions: Answer the questions based on the following information.
A road network (shown in the figure below) connects cities A, B, C and D. All road segments are straight lines. D is the mid-point on the road connecting A and C. Roads AB and BC are at right angles to each other with BC shorter than AB. The segment AB is 100 km long.
Ms X and Mr Y leave A at 8.00 a.m., take different routes to city C and reach at the same time. X takes the highway from A to B to C and travels at an average speed of 61.875 kmph. Y takes the direct route AC and travels at 45 kmph on segment AD. Y’s speed on segment DC is 55 kmph.
Question 30.
What is the length of the road segment BD?
A
50 km
B
52.5 km
C
55 km
D
Cannot be determined
Question 31.
The total distance travelled by Y during the journey is approximately
A
105 km
B
150 km
C
130 km
D
Cannot be determined
Instructions
Directions: Answer the questions based on the following information.
A road network (shown in the figure below) connects cities A, B, C and D. All road segments are straight lines. D is the mid-point on the road connecting A and C. Roads AB and BC are at right angles to each other with BC shorter than AB. The segment AB is 100 km long.
Ms X and Mr Y leave A at 8.00 a.m., take different routes to city C and reach at the same time. X takes the highway from A to B to C and travels at an average speed of 61.875 kmph. Y takes the direct route AC and travels at 45 kmph on segment AD. Y’s speed on segment DC is 55 kmph.
Directions: Answer the questions based on the following information.
A road network (shown in the figure below) connects cities A, B, C and D. All road segments are straight lines. D is the mid-point on the road connecting A and C. Roads AB and BC are at right angles to each other with BC shorter than AB. The segment AB is 100 km long.
Ms X and Mr Y leave A at 8.00 a.m., take different routes to city C and reach at the same time. X takes the highway from A to B to C and travels at an average speed of 61.875 kmph. Y takes the direct route AC and travels at 45 kmph on segment AD. Y’s speed on segment DC is 55 kmph.
Question 32.
What is the average speed of Y?
A
47.5 kmph
B
49.5 kmph
C
50 kmph
D
52 kmph
Question 33.
Navjivan Express from Ahmedabad to Chennai leaves Ahmedabad at 6.30 a.m. and travels at 50 kmph towards Baroda situated 100 km away. At 7.00 a.m. Howrah-Ahmedabad Express leaves Baroda towards Ahmedabad and travels at 40 kmph. At 7.30 a.m. Mr Shah, the traffic controller at Baroda realizes that both the trains are running on the same track. How much time does he have to avert a head-on collision between the two trains?
A
15 min
B
20 min
C
25 min
D
30 min
Instructions
Choose 1; if the question can be answered by using one of the statements alone, but cannot be answered using the other statement alone.
Choose 2; if the question can be answered by using either statement alone.
Choose 3; if the question can be answered by using both statements together, but cannot be answered using either statement alone.
Choose 4; if the question cannot be answered even by using both statements together.
Ghosh Babu has decided to take a non-stop flight from Mumbai to No-man’s-land in South America. He is scheduled to leave Mumbai at 5 am, Indian Standard Time on December 10, 2000. What is the local time at No-man’s-land when he reaches there?
- The average speed of the plane is 700 kilometres per hour.
- The flight distance is 10,500 kilometres.
Choose 1; if the question can be answered by using one of the statements alone, but cannot be answered using the other statement alone.
Choose 2; if the question can be answered by using either statement alone.
Choose 3; if the question can be answered by using both statements together, but cannot be answered using either statement alone.
Choose 4; if the question cannot be answered even by using both statements together.
Ghosh Babu has decided to take a non-stop flight from Mumbai to No-man’s-land in South America. He is scheduled to leave Mumbai at 5 am, Indian Standard Time on December 10, 2000. What is the local time at No-man’s-land when he reaches there?
- The average speed of the plane is 700 kilometres per hour.
- The flight distance is 10,500 kilometres.
Question 34.
A
1
B
2
C
3
D
4
Instructions
Answer the following question based on the information given below.
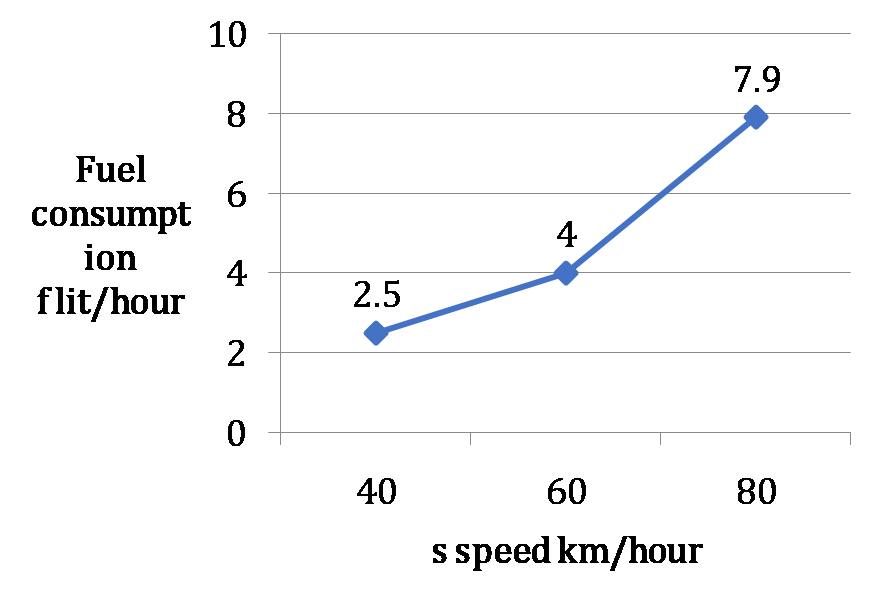
The petrol consumption rate of a new model car 'Palto' depends on its speed and may be described by the graph below
Answer the following question based on the information given below.
The petrol consumption rate of a new model car 'Palto' depends on its speed and may be described by the graph below
Question 35.
Manasa would like to minimize the fuel consumption for the trip by driving at the appropriate speed. How should she change the speed?
A
Increase the speed
B
Decrease the speed
C
Maintain the speed at 60 km/hour
D
Cannot be determined
Instructions
Answer the following question based on the information given below.
The petrol consumption rate of a new model car 'Palto' depends on its speed and may be described by the graph below
Answer the following question based on the information given below.
The petrol consumption rate of a new model car 'Palto' depends on its speed and may be described by the graph below
Question 36.
Manasa makes the 200 km trip from Mumbai to Pune at a steady speed of 60 km/hour. What is the amount of petrol consumed for the journey?
A
12.5 litres
B
13.33 litres
C
16 litres
D
19.75 litres
Question 37.
Three runners A, B and C run a race, with runner A finishing 12 metres ahead of runner B and 18 metres ahead of runner C, while runner B finishes 8 metres ahead of runner C. Each runner travels the entire distance at a constant speed. What was the length of the race?
A
36 metres
B
48 metres
C
60 metres
D
72 metres
Question 38.
A train X departs from station A at 11.00 a.m. for station B, which is 180 km away. Another train Y departs from station B at 11.00 a.m. for station A. Train X travels at an average speed of 70 km/hr and does not stop anywhere until it arrives at station B. Train Y travels at an average speed of 50 km/hr, but has to stop for 15 minutes at station C, which is 60 km away from station B enroute to station A. Ignoring the lengths of the trains, what is the distance, to the nearest km, from station A to point where the trains cross other?
A
112
B
118
C
120
D
None of these
Question 39.
Shyama and Vyom walk up an escalator (moving stairway). The escalator moves at a constant speed. Shyama takes three steps for every two of Vyom's steps. Shyama gets to the top of the escalator after having taken 25 steps. While Vyom (because his slower pace lets the escalator do a little more of the work) takes only 20 steps to reach the top. If the escalator were turned off, how many steps would they have to take to walk up?
A
40
B
50
C
60
D
80
Question 40.
At his usual rowing rate, Rahul can travel 12 miles downstream in a certain river in six hours less than it takes him to travel the same distance upstream. But if he could double his usual rowing rate for this 24 miles round trip, the downstream 12 miles would then take only one hour less than the upstream 12 miles. What is the speed of the current in miles per hour?
A
B
C
D
Question 41.
There is a tunnel connecting city A and B. There is a CAT which is standing at 3/8 the length of the tunnel from A. It listens a whistle of the train and starts running towards the entrance where, the train and the CAT meet. In another case, the CAT started running towards the exit and the train again met the CAT at the exit. What is the ratio of their speeds?
A
4 : 1
B
1 : 2
C
8 : 1
D
None of these
Question 42.
On a 20 km tunnel connecting two cities A and B there are three gutters. The distance between gutter 1 and 2 is half the distance between gutter 2 and 3. The distance from city A to its nearest gutter, gutter 1 is equal to the distance of city B from gutter 3. On a particular day the hospital in city A receives information that an accident has happened at the third gutter. The victim can be saved only if an operation is started within 40 minutes. An ambulance started from city A at 30 km/hr and crossed the first gutter after 5 minutes. If the driver had doubled the speed after that, what is the maximum amount of time the doctor would get to attend the patient at the hospital? Assume 1 minute is elapsed for taking the patient into and out of the ambulance.
A
4 minutes
B
2.5 minutes
C
1.5 minutes
D
Patient died before reaching the hospital
Question 43.
QuestionOnly a single rail track exists between station A and B on a railway line. One hour after the north bound superfast train N leaves station A for station B, a south passenger train S reaches station A from station B. The speed of the superfast train is twice that of a normal express train E, while the speed of a passenger train S is half that of E. On a particular day N leaves for station B from station A, 20 minutes behind the normal schedule. In order to maintain the schedule both N and S increased their speed. If the superfast train doubles its speed, what should be the ratio (approximately) of the speed of passenger train to that of the superfast train so that passenger train S reaches exactly at the scheduled time at the station A on that day?
A
1:3
B
1:4
C
1:5
D
1:6
Question 44.
Two straight roads R1 and R2 diverge from a point A at an angle of 120°. Ram starts walking from point A along R1 at a uniform speed of 3 km/hr. Shyam starts walking at the same time from A along R2 at a uniform speed of 2 km/hr. They continue walking for 4 hours along their respective roads and reach points B and C on R1 and R2, respectively. There is a straight line path connecting B and C. Then Ram returns to point A after walking along the line segments BC and CA. Shyam also returns to A after walking along line segments CB and BA. Their speeds remain unchanged. The time interval (in hours) between Ram’s and Shyam’s return to the point A is:
A
B
C
D
Instructions
In each question there are two statements: A and B.
Choose 1 if the question can be answered by one of the statements alone but not by the other.
Choose 2 if the question can be answered by using either statement alone.
Choose 3 if the question can be answered by using both the statements together but cannot be answered using either statement alone.
Choose 4 if the question cannot be answered even by using both the statements A and B.
If A and B run a race, then A wins by 60 seconds. If B and C run the same race, then B wins by 30 seconds. Assuming that C maintains a uniform speed, what is the time taken by C to finish the race?
A. A and C run the same race and A wins by 375 metres.
B. The length of the race is 1 km.
In each question there are two statements: A and B.
Choose 1 if the question can be answered by one of the statements alone but not by the other.
Choose 2 if the question can be answered by using either statement alone.
Choose 3 if the question can be answered by using both the statements together but cannot be answered using either statement alone.
Choose 4 if the question cannot be answered even by using both the statements A and B.
If A and B run a race, then A wins by 60 seconds. If B and C run the same race, then B wins by 30 seconds. Assuming that C maintains a uniform speed, what is the time taken by C to finish the race?
A. A and C run the same race and A wins by 375 metres.
B. The length of the race is 1 km.
Question 45.
A
1
B
2
C
3
D
4
Question 46.
In a 4000 meter race around a circular stadium having a circumference of 1000 meters, the fastest runner and the slowest runner reach the same point at the end of the 5th minute, for the first time after the start of the race. All the runners have the same starting point and each runner maintains a uniform speed throughout the race. If the fastest runner runs at twice the speed of the slowest runner, what is the time taken by the fastest runner to finish the race?
A
20 min
B
15 min
C
10 min
D
5 min
Question 47.
A sprinter starts running on a circular path of radius r metres. Her average speed (in metres/minute) is πr during the first 30 seconds, πr/2 during next one minute, πr/4 during next 2 minutes, πr/8 during next 4 minutes, and so on. What is the ratio of the time taken for the nth round to that for the previous round?
A
2
B
8
C
16
D
32
Question 48.
Two boats, travelling at 5 and 10 kms per hour, head directly towards each other. They begin at a distance of 20 kms from each other. How far apart are they (in kms) one minute before they collide?
A
B
C
D
Question 49.
If a man cycles at 10 km/hr, then he arrives at a certain place at 1 p.m. If he cycles at 15 km/hr, he will arrive at the same place at 11 a.m. At what speed must he cycle to get there at noon?
A
11 km/hr
B
12 km/hr
C
13 km/hr
D
14 km/hr
Question 50.
Karan and Arjun run a 100 metre race, where Karan beats Arjun by 10 metres. To do a favour to Arjun, Karan starts 10 metres behind the starting line in a second 100 metre race. They both run at their earlier speeds. Which of the following is true in connection with the second race?
A
Karan and Arjun reach the finishing line simultaneously.
B
Arjun beats Karan by 1 metre.
C
Arjun beats Karan by 11 metres.
D
Karan beats Arjun by 1 metre.
Instructions
Answer the next 2 questions based on the information given below.
Ram and Shyam run a race between points A and B, 5 km apart. Ram starts at 9 a.m. from A at a speed of 5 km/hr, reaches B, and returns to A at the same speed. Shyam starts at 9:45 a.m. from A at a speed of 10 km/hr, reaches B and comes back to A at the same speed.
Answer the next 2 questions based on the information given below.
Ram and Shyam run a race between points A and B, 5 km apart. Ram starts at 9 a.m. from A at a speed of 5 km/hr, reaches B, and returns to A at the same speed. Shyam starts at 9:45 a.m. from A at a speed of 10 km/hr, reaches B and comes back to A at the same speed.
Question 51.
At what time does Shyam overtake Ram?
A
10:20 a.m.
B
10:30 a.m.
C
10:40 a.m.
D
10:50 a.m.
Question 52.
At what time do Ram and Shyam first meet each other?
A
10:00 a.m.
B
10:10 a.m.
C
10:20 a.m.
D
10:30 a.m.
Question 53.
Arun, Barun and Kiranmala start from the same place and travel in the same direction at speeds of 30 km/hr, 40 km/hr and 60 km/hr respectively. Barun starts two hours after Arun. If Barun and Kiranmala overtake Arun at the same instant, how many hours after Arun did Kiranmala start?
A
3
B
3.5
C
4
D
4.5
Instructions
Answer the next 2 questions based on the information given below.
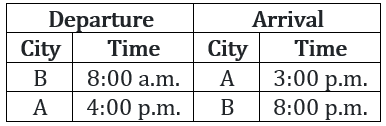
Cities A and B are in different time zones. A is located 3000 km east of B. The table below describes the schedule of an airline operating non-stop flights between A and B. All the times indicated are local and on the same day.
Assume that planes cruise at the same speed in both directions. However, the effective speed is influenced by a steady wind blowing from east to west at 50 km per hour.
Answer the next 2 questions based on the information given below.
Cities A and B are in different time zones. A is located 3000 km east of B. The table below describes the schedule of an airline operating non-stop flights between A and B. All the times indicated are local and on the same day.
Assume that planes cruise at the same speed in both directions. However, the effective speed is influenced by a steady wind blowing from east to west at 50 km per hour.
Question 54.
What is the plane’s cruising speed in km per hour?
A
700
B
550
C
600
D
500
Question 55.
What is the time difference between A and B?
A
1 hour and 30 minutes
B
2 hours
C
Cannot be determined
D
1 hour
Question 56.
Rahim plans to drive from city A to station C, at the speed of 70 km per hour, to catch a train arriving there from B. He must reach C at least 15 minutes before the arrival of the train. The train leaves B, located 500 km south of A, at 8:00 am and travels at a speed of 50 km per hour. It is known that C is located between west and northwest of B, with BC at 60° to AB. Also, C is located between south and southwest of A with AC at 30° to AB. The latest time by which Rahim must leave A and still catch the train is closest to
A
6:15 am
B
6:30 am
C
6:45 am
D
7:00 am
Question 57.
A motorbike leaves point A at 1 pm and moves towards point B at a uniform speed. A car leaves point B at 2 pm and moves towards point A at a uniform speed which is double that of the motorbike. They meet at 3:40 pm at a point which is 168 km away from A. What is the distance, in km, between A and B?
A
364
B
378
C
380
D
388
Question 58.
Arun drove from home to his hostel at 60 miles per hour. While returning home he drove half way along the same route at a speed of 25 miles per hour and then took a bypass road which increased his driving distance by 5 miles, but allowed him to drive at 50 miles per hour along this bypass road. If his return journey took 30 minutes more than his onward journey, then the total distance traveled by him is
A
55 miles
B
60 miles
C
65 miles
D
70 miles
Question 59.
In a 10 km race, A, B and C, each running at uniform speed, get the gold, silver, and bronze medals, respectively. If A beats B by 1 km and B beats C by 1 km, then by how many metres does A beat C?
A
B
C
D
Question 60.
A man travels by a motor boat down a river to his office and back. With the speed of the river unchanged, if he doubles the speed of his motor boat, then his total travel time gets reduced by 75%. The ratio of the original speed of the motor boat to the speed of the river is:
A
√6 : √2
B
√7 : 2
C
2√5 : 3
D
3 : 2
Question 61.
A man leaves his home and walks at a speed of 12 km per hour, reaching the railway station 10 minutes after the train had departed. If instead he had walked at a speed of 15 km per hour, he would have reached the station 10 minutes before the train's departure. The distance (in km) from his home to the railway station is:
A
B
C
D
Question 62.
Points A, P, Q and B lie on the same line such that P, Q and B are, respectively, 100 km, 200 km and 300 km away from A. Cars 1 and 2 leave A at the same time and move towards B. Simultaneously, car 3 leaves B and moves towards A. Car 3 meets car 1 at Q, and car 2 at P. If each car is moving in uniform speed then the ratio of the speed of car 2 to that of car 1 is
A
1:2
B
2:7
C
1:4
D
2:9
Question 63.
Points A and B are 150 km apart. Cars 1 and 2 travel from A to B, but car 2 starts from A when car 1 is already 20 km away from A. Each car travels at a speed of 100 kmph for the first 50 km, at 50 kmph for the next 50 km, and at 25 kmph for the last 50 km. The distance, in km, between car 2 and B when car 1 reaches B is
A
B
C
D
Question 64.
On a long stretch of east-west road, A and B are two points such that B is 350 km west of A. One car starts from A and another from B at the same time. If they move towards each other, then they meet after 1 hour. If they both move towards east, then they meet in 7 hrs. The difference between their speeds, in km per hour, is
A
B
C
D
Question 65.
The distance from A to B is 60 km. Partha and Narayan start from A at the same time and move towards B. Partha takes four hours more than Narayan to reach B. Moreover, Partha reaches the mid-point of A and B two hours before Narayan reaches B. The speed of Partha, in km per hour, is
A
4
B
3
C
6
D
5
Question 66.
Point P lies between points A and B such that the length of BP is thrice that of AP. Car 1 starts from A and moves towards B. Simultaneously, car 2 starts from B and moves towards A. Car 2 reaches P one hour after car 1 reaches P. If the speed of car 2 is half that of car 1, then the time, in minutes, taken by car 1 in reaching P from A is
A
B
C
D
Question 67.
Train T leaves station X for station Y at 3 pm. Train S, traveling at three quarters of the speed of T, leaves Y for X at 4 pm. The two trains pass each other at a station Z, where the distance between X and Z is three-fifths of that between X and Y. How many hours does train T take for its journey from X to Y?
A
B
C
D
Question 68.
Two ants A and B start from a point P on a circle at the same time, with A moving clock-wise and B moving anti-clockwise. They meet for the first time at 10:00 am when A has covered 60% of the track. If A returns to P at 10:12 am, then B returns to P at
A
10:27 am
B
10:25 am
C
10:45 am
D
10:18 am
Question 69.
John jogs on track A at 6 kmph and Mary jogs on track B at 7.5 kmph. The total length of tracks A and B is 325 metres. While John makes 9 rounds of track A, Mary makes 5 rounds of track B. In how many seconds will Mary make one round of track A?
A
B
C
D
Question 70.
A cyclist leaves A at 10 am and reaches B at 11 am. Starting from 10:01 am, every minute a motorcycle leaves A and moves towards B. Forty-five such motorcycles reach B by 11 am. All motorcycles have the same speed. If the cyclist had doubled his speed, how many motorcycles would have reached B by the time the cyclist reached B?
A
22
B
20
C
15
D
23
Question 71.
The wheels of bicycles A and B have radii 30 cm and 40 cm, respectively. While traveling a certain distance, each wheel of A required 5000 more revolutions than each wheel of B. If bicycle B traveled this distance in 45 minutes, then its speed, in km per hour, was
A
14π
B
18π
C
16π
D
12π
Question 72.
In a race of three horses, the first beat the second by 11 metres and the third by 90 metres. If the second beat the third by 80 metres, what was the length, in metres, of the racecourse?
A
B
C
D
Question 73.
One can use three different transports which move at 10, 20, and 30 kmph, respectively. To reach from A to B, Amal took each mode of transport for 1/3 of his total journey time, while Bimal took each mode of transport for 1/3 of the total distance. The percentage by which Bimal’s travel time exceeds Amal’s travel time is nearest to
A
22
B
21
C
20
D
19
Question 74.
Two cars travel the same distance starting at 10:00 am and 11:00 am, respectively, on the same day. They reach their common destination at the same point of time. If the first car travelled for at least 6 hours, then the highest possible value of the percentage by which the speed of the second car could exceed that of the first car is
A
25
B
10
C
30
D
20
Question 75.
Anil, Sunil, and Ravi run along a circular path of length 3 km, starting from the same point at the same time, and going in the clockwise direction. If they run at speeds of 15km/hr, 10 km/hr, and 8 km/hr, respectively, how much distance in km will Ravi have run when Anil and Sunil meet again for the first time at the starting point?
A
4.6
B
4.2
C
5.2
D
4.8
Question 76.
Vimla starts for office every day at 9 am and reaches exactly on time if she drives at her usual speed of 40 km/hr. She is late by 6 minutes if she drives at 35 km/hr. One day, she covers two-thirds of her distance to office in one-thirds of her usual time to reach office, and then stops for 8 minutes. The speed, in km/hr, at which she should drive the remaining distance to reach office exactly on time is
A
26
B
28
C
29
D
27
Question 77.
A and B are two railway stations 90 km apart. A train leaves A at 9:00 am, heading towards B at a speed of 40 km/hr. Another train leaves B at 10:30 am, heading towards A at a speed of 20 km/hr. The trains meet each other at
A
11:45 am
B
11 am
C
10:45 am
D
11:20 am
Question 78.
The distance from B to C is thrice that from A to B. Two trains travel from A to C via B. The speed of train 2 is double that of train 1 while traveling from A to B and their speeds are interchanged while traveling from B to C. The ratio of the time taken by train 1 to that taken by train 2 in travelling from A to C is
A
4:1
B
7:5
C
5:7
D
1:4
Question 79.
Two circular tracks T1 and T2 of radii 100 m and 20 m, respectively touch at a point A. Starting from A at the same time, Ram and Rahim are walking on track T1 and track T2 at speeds 15 km/hr and 5 km/hr respectively. The number of full rounds that Ram will make before he meets Rahim again for the first time is
A
3
B
5
C
4
D
2
Question 80.
A and B are two points on a straight line. Ram runs from A to B while Rahim runs from B to A. After crossing each other, Ram and Rahim reach their destinations in one minute and four minutes, respectively. If they start at the same time, then the ratio of Ram's speed to Rahim's speed is
A
√2
B
2√2
C
1/2
D
2
Question 81.
In a car race, car A beats car B by 45 km, car B beats car C by 50 km, and car A beats car C by 90 km. The distance (in km) over which the race has been conducted is
A
550
B
450
C
475
D
500
Question 82.
A train travelled at one-thirds of its usual speed, and hence reached the destination 30 minutes after the scheduled time. On its return journey, the train initially travelled at its usual speed for 5 minutes but then stopped for 4 minutes for an emergency. The percentage by which the train must now increase its usual speed so as to reach the destination at the scheduled time, is nearest to
A
58
B
61
C
67
D
50
Question 83.
Leaving home at the same time, Amal reaches office at 10:15 am if he travels at 8 km/hr, and at 9:40 am if he travels at 15 km/hr. Leaving home at 9:10 am, at what speed, in km/hr, must he travel so as to reach office exactly at 10 am?
A
13
B
14
C
11
D
12
Question 84.
A straight road connects points A and B. Car 1 travels from A to B and Car 2 travels from B to A, both leaving at the same time. After meeting each other, they take 45 minutes and 20 minutes, respectively, to complete their journeys. If Car 1 travels at the speed of 60 km/hr, then the speed of Car 2, in km/hr, is
A
100
B
90
C
70
D
80
Question 85.
Two persons are walking beside a railway track at respective speeds of 2 and 4 km per hour in the same direction. A train came from behind them and crossed them in 90 and 100 seconds, respectively. The time, in seconds, taken by the train to cross an electric post is nearest to
A
75
B
82
C
87
D
78
Question 86.
Mira and Amal walk along a circular track, starting from the same point at the same time. If they walk in the same direction, then in 45 minutes, Amal completes exactly 3 more rounds than Mira. If they walk in opposite directions, then they meet for the first time exactly after 3 minutes. The number of rounds Mira walks in one hour is
A
B
C
D
Question 87.
Two trains A and B were moving in opposite directions, their speeds being in the ratio 5 : 3. The front end of A crossed the rear end of B 46 seconds after the front ends of the trains had crossed each other. It took another 69 seconds for the rear ends of the trains to cross each other. The ratio of length of train A to that of train B is
A
2:1
B
3:2
C
2:3
D
5:3
Question 88.
Two trains cross each other in 14 seconds when running in opposite directions along parallel tracks. The faster train is 160 m long and crosses a lamp post in 12 seconds. If the speed of the other train is 6 km/hr less than the faster one, its length, in m, is
A
180
B
184
C
190
D
192
Question 89.
Two cars travel from different locations at constant speeds. To meet each other after starting at the same time, they take 1.5 hours if they travel towards each other, but 10.5 hours if they travel in the same direction. If the speed of the slower car is 60 km/hr, then the distance travelled, in km, by the slower car when it meets the other car while traveling towards each other, is
A
90
B
120
C
100
D
150
Question 90.
Moody takes 30 seconds to finish riding an escalator if he walks on it at his normal speed in the same direction. He takes 20 seconds to finish riding the escalator if he walks at twice his normal speed in the same direction. If Moody decides to stand still on the escalator, then the time, in seconds, needed to finish riding the escalator is
A
B
C
D
Question 91.
Two ships meet mid-ocean, and then, one ship goes south and the other ship goes west, both travelling at constant speeds. Two hours later, they are 60 km apart. If the speed of one of the ships is 6 km per hour more than the other one, then the speed, in km per hour, of the slower ship is
A
18
B
24
C
12
D
20
Question 92.
Trains A and B start traveling at the same time towards each other with constant speeds from stations X and Y, respectively. Train A reaches station Y in 10 minutes while train B takes 9 minutes to reach station X after meeting train A. Then the total time taken, in minutes, by train B to travel from station Y to station X is
A
12
B
15
C
10
D
6
Question 93.
A boat takes 2 hours to travel downstream a river from port A to port B, and 3 hours to return to port A. Another boat takes a total of 6 hours to travel from port B to port A and return to port B. If the speeds of the boats and the river are constant, then the time, in hours, taken by the slower boat to travel from port A to port B is?
A
3(3 + √5)
B
3(√5 - 1)
C
3(3 - √5)
D
12(√5 - 2)
Question 94.
Ravi is driving at a speed of 40 km/h on a road. Vijay is 54 meters behind Ravi and driving in the same direction as Ravi. Ashok is driving along the same road from the opposite direction at a speed of 50 km/h and is 225 meters away from Ravi. The speed, in km/h, at which Vijay should drive so that all the three cross each other at the same time, is
A
67.2
B
58.8
C
61.6
D
64.4
Question 95.
Arvind travels from town A to town B, and Surbhi from town B to town A, both starting at the same time along the same route. After meeting each other, Arvind takes 6 hours to reach town B while Surbhi takes 24 hours to reach town A. If Arvind travelled at a speed of 54 km/h, then the distance, in km, between town A and town B is
A
B
C
D
Question 96.
Brishti went on an 8-hour trip in a car. Before the trip, the car had travelled a total of x km till then, where x is a whole number and is palindromic, i.e., x remains unchanged when its digits are reversed. At the end of the trip, the car had travelled a total of 26862 kms till then, this number again being palindromic. If Brishti never drove at more than 110 kmph, then the greatest possible average speed at which she drove during the rip, in kmph was?
A
110
B
80
C
90
D
100
