| Test Name | No. of Questions | Marks (of each) | Time | Take Test |
|---|---|---|---|---|
| Test-1 | 14 | 3 | 40 Minutes | |
| Test-2 | 14 | 3 | 40 Minutes | |
| Test-3 | 14 | 3 | 40 Minutes | |
| Test-4 | 14 | 3 | 40 Minutes | |
| Test-5 | 17 | 3 | 40 Minutes |
Question 1.
Manu earns ₹4000 per month and wants to save an average of ₹550 per month in a year. In the first nine months, his monthly expense was ₹3500, and he foresees that, tenth month onward, his monthly expense will increase to ₹3700. In order to meet his yearly savings target, his monthly earnings, in rupees, from the tenth month onward should be:
Instructions
Direction: Answer the questions based on the following information.
Four sisters — Suvarna, Tara, Uma and Vibha are playing a game such that the loser doubles the money of each of the other players from her share. They played four games and each sister lost one game in alphabetical order. At the end of fourth game, each sister had Rs.32.
Direction: Answer the questions based on the following information.
Four sisters — Suvarna, Tara, Uma and Vibha are playing a game such that the loser doubles the money of each of the other players from her share. They played four games and each sister lost one game in alphabetical order. At the end of fourth game, each sister had Rs.32.
Question 2.
How many rupees did Suvarna start with?
Instructions
Answer the question based on the following information.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
Answer the question based on the following information.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
Question 3.
If Roopa leaves home with 30 flowers, the number of flowers she offers to each deity is
Instructions
Each question is followed by two statements, A and B. Answer each question using the following instructions
Choose 1 if the question can be answered by using one of the statements alone but not by using the other statement alone.
Choose 2 if the question can be answered by using either of the statements alone.
Choose 3 if the question can be answered by using both statements together but not by either statement alone.
Choose 4 if the question cannot be answered on the basis of the two statements.
Nandini paid for an article using currency notes of denominations Re. l, Rs. 2, Rs. 5, and Rs. 10 using at least one note of each denomination. The total number of five and ten rupee notes used was one more than the total number of one and two rupee notes used. What was the price of the article?
A. Nandini used a total of 13 currency notes.
B. The price of the article was a multiple of Rs. 10.
Each question is followed by two statements, A and B. Answer each question using the following instructions
Choose 1 if the question can be answered by using one of the statements alone but not by using the other statement alone.
Choose 2 if the question can be answered by using either of the statements alone.
Choose 3 if the question can be answered by using both statements together but not by either statement alone.
Choose 4 if the question cannot be answered on the basis of the two statements.
Nandini paid for an article using currency notes of denominations Re. l, Rs. 2, Rs. 5, and Rs. 10 using at least one note of each denomination. The total number of five and ten rupee notes used was one more than the total number of one and two rupee notes used. What was the price of the article?
A. Nandini used a total of 13 currency notes.
B. The price of the article was a multiple of Rs. 10.
Question 4.
Question 5.
Gopal went to a fruit market with certain amount of money. With this money he can buy either 50 oranges or 40 mangoes. He retains 10% of the money for taxi fare. If he buys 20 mangoes, then the number of oranges he can buy is
Instructions
Answer the question based on the following information.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
Answer the question based on the following information.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
Question 6.
The minimum number of flowers that could be offered to each deity is
Question 7.
Five students, including Amit, appear for an examination in which possible marks are integers between 0 and 50, both inclusive. The average marks for all the students is 38 and exactly three students got more than 32. If no two students got the same marks and Amit got the least marks among the five students, then the difference between the highest and lowest possible marks of Amit is
Instructions
Answer the following question based on the information given below.
A certain perfume is available at a duty-free shop at the Bangkok international airport. It is priced in the Thai currency Baht but other currencies are also acceptable. In particular, the shop accepts Euro and US Dollar at the following rates of exchange:
US Dollar 1 = 41 Bahts and Euro 1 = 46 Bahts
The perfume is priced at 520 Bahts per bottle. After one bottle is purchased, subsequent bottles are available at a discount of 30%. Three friends S, R and M together purchase three bottles of the perfume, agreeing to share the cost equally. R pays 2 Euros. M pays 4 Euros and 27 Thai Bahts and S pays the remaining amount in US Dollars.
Answer the following question based on the information given below.
A certain perfume is available at a duty-free shop at the Bangkok international airport. It is priced in the Thai currency Baht but other currencies are also acceptable. In particular, the shop accepts Euro and US Dollar at the following rates of exchange:
US Dollar 1 = 41 Bahts and Euro 1 = 46 Bahts
The perfume is priced at 520 Bahts per bottle. After one bottle is purchased, subsequent bottles are available at a discount of 30%. Three friends S, R and M together purchase three bottles of the perfume, agreeing to share the cost equally. R pays 2 Euros. M pays 4 Euros and 27 Thai Bahts and S pays the remaining amount in US Dollars.
Question 8.
How much does R owe to S in Thai Baht?
Instructions
Direction: Answer the questions based on the following information.
Four sisters — Suvarna, Tara, Uma and Vibha are playing a game such that the loser doubles the money of each of the other players from her share. They played four games and each sister lost one game in alphabetical order. At the end of fourth game, each sister had Rs.32.
Direction: Answer the questions based on the following information.
Four sisters — Suvarna, Tara, Uma and Vibha are playing a game such that the loser doubles the money of each of the other players from her share. They played four games and each sister lost one game in alphabetical order. At the end of fourth game, each sister had Rs.32.
Question 9.
Who started with the lowest amount?
Question 10.
Three times the first of three consecutive odd integers is 3 more than twice the third. What is the third integer?
Instructions
Answer the following question based on the information given below.
A certain perfume is available at a duty-free shop at the Bangkok international airport. It is priced in the Thai currency Baht but other currencies are also acceptable. In particular, the shop accepts Euro and US Dollar at the following rates of exchange:
US Dollar 1 = 41 Bahts and Euro 1 = 46 Bahts
The perfume is priced at 520 Bahts per bottle. After one bottle is purchased, subsequent bottles are available at a discount of 30%. Three friends S, R and M together purchase three bottles of the perfume, agreeing to share the cost equally. R pays 2 Euros. M pays 4 Euros and 27 Thai Bahts and S pays the remaining amount in US Dollars.
Answer the following question based on the information given below.
A certain perfume is available at a duty-free shop at the Bangkok international airport. It is priced in the Thai currency Baht but other currencies are also acceptable. In particular, the shop accepts Euro and US Dollar at the following rates of exchange:
US Dollar 1 = 41 Bahts and Euro 1 = 46 Bahts
The perfume is priced at 520 Bahts per bottle. After one bottle is purchased, subsequent bottles are available at a discount of 30%. Three friends S, R and M together purchase three bottles of the perfume, agreeing to share the cost equally. R pays 2 Euros. M pays 4 Euros and 27 Thai Bahts and S pays the remaining amount in US Dollars.
Question 11.
How much does M owe to S in US Dollars?
Instructions
Direction: Answer the questions based on the following information.
Four sisters — Suvarna, Tara, Uma and Vibha are playing a game such that the loser doubles the money of each of the other players from her share. They played four games and each sister lost one game in alphabetical order. At the end of fourth game, each sister had Rs.32.
Direction: Answer the questions based on the following information.
Four sisters — Suvarna, Tara, Uma and Vibha are playing a game such that the loser doubles the money of each of the other players from her share. They played four games and each sister lost one game in alphabetical order. At the end of fourth game, each sister had Rs.32.
Question 12.
Who started with the highest amount?
Instructions
Answer the question based on the following information.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
Answer the question based on the following information.
A young girl Roopa leaves home with x flowers, goes to the bank of a nearby river. On the bank of the river, there are four places of worship, standing in a row. She dips all the x flowers into the river. The number of flowers doubles. Then she enters the first place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the second place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the third place of worship, offers y flowers to the deity. She dips the remaining flowers into the river, and again the number of flowers doubles. She goes to the fourth place of worship, offers y flowers to the deity. Now she is left with no flowers in hand.
Question 13.
The minimum number of flowers with which Roopa leaves home is
Question 14.
In an examination, there were 75 questions. 3 marks were awarded for each correct answer, 1 mark was deducted for each wrong answer and 1 mark was awarded for each unattempted question. Rayan scored a total of 97 marks in the examination. If the number of unattempted questions was higher than the number of attempted questions, then the maximum number of correct answers that Rayan could have given in the examination is:
Question 15.
Iqbal dealt some cards to Mushtaq and himself from a full pack of playing cards and laid the rest aside. Iqbal then said to Mushtaq. “If you give me a certain number of your cards, I will have four times as many cards as you will have. If I give you the same number of cards, I will have thrice as many cards as you will have “. Of the given choices, which could represent the number of cards with Iqbal?
Question 16.
A donation box can receive only cheques of ₹100, ₹250, and ₹500. On one good day, the donation box was found to contain exactly 100 cheques amounting to a total sum of ₹15250. Then, the maximum possible number of cheques of ₹500 that the donation box may have contained, is
Instructions
Which one of the following conditions must p, q and r satisfy so that the following system of linear simultaneous equations has at least one solution, such that p + q + r ≠ 0?
x + 2y − 3z = p
2x + 6y − 11z = q
x − 2y + 7z = r
Which one of the following conditions must p, q and r satisfy so that the following system of linear simultaneous equations has at least one solution, such that p + q + r ≠ 0?
x + 2y − 3z = p
2x + 6y − 11z = q
x − 2y + 7z = r
Question 17.
Question 18.
What is the value of k for which the following system of equations has no solution:2x – 8y = 3 and kx +4y = 10
Instructions
Directions: Answer the questions based on the following information.
Rajiv reaches city B from city A in 4 hours, driving at speed of 35 kmph for the first two hour and at 45 kmph for the next two hours. Aditi follows the same route, but drives at three different speeds: 30, 40 and 50 kmph, covering an equal distance in each speed segment. The two cars are similar with petrol consumption characteristics (km per litre) shown in the figure below.
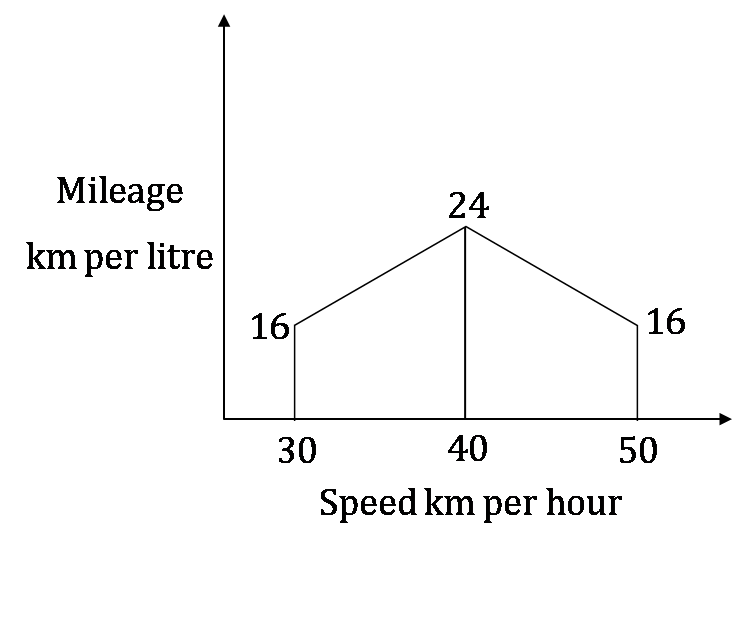
Directions: Answer the questions based on the following information.
Rajiv reaches city B from city A in 4 hours, driving at speed of 35 kmph for the first two hour and at 45 kmph for the next two hours. Aditi follows the same route, but drives at three different speeds: 30, 40 and 50 kmph, covering an equal distance in each speed segment. The two cars are similar with petrol consumption characteristics (km per litre) shown in the figure below.

Question 19.
The quantity of petrol consumed by Aditi for the journey is
Instructions
Direction: Answer the questions based on the following information.
Four sisters — Suvarna, Tara, Uma and Vibha are playing a game such that the loser doubles the money of each of the other players from her share. They played four games and each sister lost one game in alphabetical order. At the end of fourth game, each sister had Rs.32.
Direction: Answer the questions based on the following information.
Four sisters — Suvarna, Tara, Uma and Vibha are playing a game such that the loser doubles the money of each of the other players from her share. They played four games and each sister lost one game in alphabetical order. At the end of fourth game, each sister had Rs.32.
Question 20.
What was the amount with Uma at the end of the second round?
Question 21.
John bought five mangoes and ten oranges together for forty rupees. Subsequently, he returned one mango and got two oranges in exchange. The price of an orange would be
Question 22.
A basket of 2 apples, 4 oranges and 6 mangoes costs the same as a basket of 1 apple, 4 oranges and 8 mangoes, or a basket of 8 oranges and 7 mangoes. Then the number of mangoes in a basket of mangoes that has the same cost as the other baskets is
Instructions
Directions: Answer the questions based on the following information.
Rajiv reaches city B from city A in 4 hours, driving at speed of 35 kmph for the first two hour and at 45 kmph for the next two hours. Aditi follows the same route, but drives at three different speeds: 30, 40 and 50 kmph, covering an equal distance in each speed segment. The two cars are similar with petrol consumption characteristics (km per litre) shown in the figure below.

Directions: Answer the questions based on the following information.
Rajiv reaches city B from city A in 4 hours, driving at speed of 35 kmph for the first two hour and at 45 kmph for the next two hours. Aditi follows the same route, but drives at three different speeds: 30, 40 and 50 kmph, covering an equal distance in each speed segment. The two cars are similar with petrol consumption characteristics (km per litre) shown in the figure below.

Question 23.
Zoheb would like to drive Aditi’s car over the same route from A to B and minimize the petrol consumption for the trip. What is the quantity of petrol required by him?
Question 24.
A test has 50 questions. A student scores 1 mark for a correct answer, −1/3 for a wrong answer, and −1/6 for not attempting a question. If the net score of a student is 32, the number of questions answered wrongly by that student cannot be less than
Instructions
Direction: Each question is followed by two statements, I and II. Answer the questions based on the statements and mark the answer as
1. if the question can be answered with the help of any one statement alone but not by the other statement.
2. if the question can be answered with the help of either of the statements taken individually.
3. if the question can be answered with the help of both statements together.
4. if the question cannot be answered even with the help of both statements together.
What is the price of tea?
I. Price of coffee is Rs. 5 more than that of tea.
II. Price of coffee is Rs. 5 less than the price of a cold drink which cost three times the price of tea.
Direction: Each question is followed by two statements, I and II. Answer the questions based on the statements and mark the answer as
1. if the question can be answered with the help of any one statement alone but not by the other statement.
2. if the question can be answered with the help of either of the statements taken individually.
3. if the question can be answered with the help of both statements together.
4. if the question cannot be answered even with the help of both statements together.
What is the price of tea?
I. Price of coffee is Rs. 5 more than that of tea.
II. Price of coffee is Rs. 5 less than the price of a cold drink which cost three times the price of tea.
Question 25.
Instructions
Use the following information:
Eighty five children went to an amusement park where they could ride on the merry – go round, roller coaster, and Ferris wheel. It was known that 20 of them took all three rides, and 55 of them took at least two of the three rides. Each ride cost Re.1, and the total receipt of the amusement park was Rs.145.
Use the following information:
Eighty five children went to an amusement park where they could ride on the merry – go round, roller coaster, and Ferris wheel. It was known that 20 of them took all three rides, and 55 of them took at least two of the three rides. Each ride cost Re.1, and the total receipt of the amusement park was Rs.145.
Question 26.
How many children did not try any of the rides?
Instructions
A survey on a sample of 25 new cars being sold at a local auto dealer was conducted to see which of the three popular options- air conditioning, radio and power windows- were already installed. The survey found
- 15 had air conditioning,
- 2 had air conditioning and power windows but no radios,
- 12 had radio,
- 6 had air conditioning and radio, but no power windows,
- 11 had power windows,
- 4 had radio and power windows and
- 3 had all three options.
What is the number of cars that had none of the options?
A survey on a sample of 25 new cars being sold at a local auto dealer was conducted to see which of the three popular options- air conditioning, radio and power windows- were already installed. The survey found
- 15 had air conditioning,
- 2 had air conditioning and power windows but no radios,
- 12 had radio,
- 6 had air conditioning and radio, but no power windows,
- 11 had power windows,
- 4 had radio and power windows and
- 3 had all three options.
What is the number of cars that had none of the options?
Question 27.
Question 28.
How many three-digit numbers are greater than 100 and increase by 198 when the three digits are arranged in the reverse order
Question 29.
A thief was stealing diamonds from a jewellery store. On his way out, he encountered three guards, each was given half of the existing diamonds and two over it by the thief. In the end, he was left with one diamond. How many did the thief steal?
Question 30.
Once I had been to the post office to buy five-rupee, two-rupee and one-rupee stamps. I paid the clerk Rs. 20, and since he had no change, he gave me three more one-rupee stamps. If the number of stamps of each type that I had ordered initially was more than one, what was the total number of stamps that I bought?
Question 31.
A box has 450 balls, each either white or black, there being as many metallic white balls as metallic black balls. If 40% of the white balls and 50% of the black balls are metallic, then the number of non-metallic balls in the box is
Question 32.
Using only 2, 5, 10, 25 and 50 paise coins, what will be the minimum number of coins required to pay exactly 78 paise, 69 paise and Rs 1.01 to three different persons?
Question 33.
Amar went for a holiday to his friend's place. They together either went for yoga in the morning or played tennis in the evening but not both. 14 mornings and 24 evenings, they both stayed home and they both went out together for 22 days. How many days did Amar stay at his friend's place?
Instructions
A salesman enters the quantity sold and the price into the computer. Both the numbers are two-digit numbers. But, by mistake, both the numbers were entered with their digits interchanged. The total sales value remained the same, i.e. Rs. 1,148, but the inventory reduced by 54.
A salesman enters the quantity sold and the price into the computer. Both the numbers are two-digit numbers. But, by mistake, both the numbers were entered with their digits interchanged. The total sales value remained the same, i.e. Rs. 1,148, but the inventory reduced by 54.
Question 34.
What is the actual price per piece?
Instructions
Use the following information:
Eighty five children went to an amusement park where they could ride on the merry – go round, roller coaster, and Ferris wheel. It was known that 20 of them took all three rides, and 55 of them took at least two of the three rides. Each ride cost Re.1, and the total receipt of the amusement park was Rs.145.
Use the following information:
Eighty five children went to an amusement park where they could ride on the merry – go round, roller coaster, and Ferris wheel. It was known that 20 of them took all three rides, and 55 of them took at least two of the three rides. Each ride cost Re.1, and the total receipt of the amusement park was Rs.145.
Question 35.
How many children took exactly one ride?
Question 36.
and = x, then
Question 37.
If x and y are integers then the equation 5x + 19y = 64 has:
Question 38.
A gentleman decided to treat a few children in the following manner. He gives half of his total stock of toffees and one extra to the first child, and then the half of the remaining stock along with one extra to the second and continues giving away in this fashion. His total stock exhausts after he takes care of 5 children. How many toffees were there in his stock initially?
Question 39.
Two oranges, three bananas and four apples cost Rs.15. Three oranges, two bananas and one apple cost Rs 10. I bought 3 oranges, 3 bananas and 3 apples. How much did I pay?
Instructions
A salesman enters the quantity sold and the price into the computer. Both the numbers are two-digit numbers. But, by mistake, both the numbers were entered with their digits interchanged. The total sales value remained the same, i.e. Rs. 1,148, but the inventory reduced by 54.
A salesman enters the quantity sold and the price into the computer. Both the numbers are two-digit numbers. But, by mistake, both the numbers were entered with their digits interchanged. The total sales value remained the same, i.e. Rs. 1,148, but the inventory reduced by 54.
Question 40.
What is the actual quantity sold?
Question 41.
Aron bought some pencils and sharpeners. Spending the same amount of money as Aron, Aditya bought twice as many pencils and 10 less sharpeners. If the cost of one sharpener is ₹ 2 more than the cost of a pencil, then the minimum possible number of pencils bought by Aron and Aditya together is
Question 42.
The points of intersection of three lines 2X + 3Y – 5 = 0, 5X – 7Y + 2 = 0 and 9X – 5Y – 4= 0
Instructions
A string of three English letters is formed as per the following rules:
- The first letter is any vowel.
- The second letter is m, n or p.
- If the second letter is m, then the third letter is any vowel which is different from the first letter.
- If the second letter is n, then the third letter is e or u.
- If the second letter is p, then the third letter is the same as the first letter.
A string of three English letters is formed as per the following rules:
- The first letter is any vowel.
- The second letter is m, n or p.
- If the second letter is m, then the third letter is any vowel which is different from the first letter.
- If the second letter is n, then the third letter is e or u.
- If the second letter is p, then the third letter is the same as the first letter.
Question 43.
How many strings of letters can possibly be formed using the above rules?
Question 44.
Every ten years the Indian government counts all the people living in the country. Suppose that the director of the census has reported the following data on two neighbouring villages Chota hazri and Mota hazri:Chota hazri has 4,522 fewer males than Mota hazri. Mota hazri has 4,020 more females than males. Chota hazri has twice as many females as males. Chota hazri has 2,910 fewer females than Mota hazri. What is the total number of males in Chota hazri?
Instructions
Data is provided followed by two statements – I and II – both resulting in a value, say I and II.
As your answer,
Type 1, if I > II.
Type 2, if I < II.
Type 3, if I = II.
Type 4, if nothing can be said.
Data is provided followed by two statements – I and II – both resulting in a value, say I and II.
As your answer,
Type 1, if I > II.
Type 2, if I < II.
Type 3, if I = II.
Type 4, if nothing can be said.
Question 45.
Last week Martin received 5 per week plus a commission of 2 cents for each of the first 25 copies sold, 3 cents for each of next 25 copies sold and 4 cents for each copy thereafter. ($1 = 100 cents). I. Martin’s commission in the last week. II. Miguel’s total income for last week.
Question 46.
At a certain fast food restaurant, Brian can buy 3 burgers, 7 shakes, and one order of fries for Rs. 120 exactly. At the same place it would cost Rs. 164.5 for 4 burgers, 10 shakes, and one order of fries. How much would it cost for an ordinary meal of one burger, one shake, and one order of fries?
Instructions
Directions: Answer the questions based on the following information.
Recently, Ghosh Babu spent his winter vacation on Kyakya Island. During the vacation, he visited the local casino where he came across a new card game. Two players, using a normal deck of 52 playing cards, play this game. One player is called the ‘dealer’ and the other is called the ‘player’. First, the player picks a card at random from the deck. This is called the base card. The amount in rupees equal to the face value of the base card is called the base amount. The face values of ace, king, queen and jack are ten. For other cards the face value is the number on the card. Once the ‘player’ picks a card from the deck, the ‘dealer’ pays him the base amount. Then the ‘dealer’ picks a card from the deck and this card is called the top card. If the top card is of the same suit as the base card, the ‘player’ pays twice the base amount to the ‘dealer’. If the top card is of the same colour as the base card (but not the same suit), then the ‘player’ pays the base amount to the ‘dealer’. If the top card happens to be of a different colour than the base card, the ‘dealer’ pays the base amount to the ‘player’.
Ghosh Babu played the game four times. First time he picked eight of clubs and the ‘dealer’ picked queen of clubs. Second time, he picked ten of hearts and the ‘dealer’ picked two of spades. Next time, Ghosh Babu picked six of diamonds and the ‘dealer’ picked ace of hearts. Lastly, he picked eight of spades and the ‘dealer’ picked jack of spades. Answer the following questions based on these four games.
Directions: Answer the questions based on the following information.
Recently, Ghosh Babu spent his winter vacation on Kyakya Island. During the vacation, he visited the local casino where he came across a new card game. Two players, using a normal deck of 52 playing cards, play this game. One player is called the ‘dealer’ and the other is called the ‘player’. First, the player picks a card at random from the deck. This is called the base card. The amount in rupees equal to the face value of the base card is called the base amount. The face values of ace, king, queen and jack are ten. For other cards the face value is the number on the card. Once the ‘player’ picks a card from the deck, the ‘dealer’ pays him the base amount. Then the ‘dealer’ picks a card from the deck and this card is called the top card. If the top card is of the same suit as the base card, the ‘player’ pays twice the base amount to the ‘dealer’. If the top card is of the same colour as the base card (but not the same suit), then the ‘player’ pays the base amount to the ‘dealer’. If the top card happens to be of a different colour than the base card, the ‘dealer’ pays the base amount to the ‘player’.
Ghosh Babu played the game four times. First time he picked eight of clubs and the ‘dealer’ picked queen of clubs. Second time, he picked ten of hearts and the ‘dealer’ picked two of spades. Next time, Ghosh Babu picked six of diamonds and the ‘dealer’ picked ace of hearts. Lastly, he picked eight of spades and the ‘dealer’ picked jack of spades. Answer the following questions based on these four games.
Question 47.
If Ghosh Babu stopped playing the game when his gain would be maximized, the gain in Rs. would have been
Question 48.
Let k be a constant. The equations kx + y = 3 and 4x + ky = 4 have a unique solution if and only if
Instructions
Data is provided followed by two statements – I and II – both resulting in a value, say I and II.
As your answer,
Type 1, if I > II.
Type 2, if I < II.
Type 3, if I = II.
Type 4, if nothing can be said.
Nineteen years from now Jackson will be 3 times as old as Joseph is now. Johnson is three years younger than Jackson.
I. Johnson’s age now.
II. Joseph’s age now.
Data is provided followed by two statements – I and II – both resulting in a value, say I and II.
As your answer,
Type 1, if I > II.
Type 2, if I < II.
Type 3, if I = II.
Type 4, if nothing can be said.
Nineteen years from now Jackson will be 3 times as old as Joseph is now. Johnson is three years younger than Jackson.
I. Johnson’s age now.
II. Joseph’s age now.
Question 49.
Instructions
A string of three English letters is formed as per the following rules:
- The first letter is any vowel.
- The second letter is m, n or p.
- If the second letter is m, then the third letter is any vowel which is different from the first letter.
- If the second letter is n, then the third letter is e or u.
- If the second letter is p, then the third letter is the same as the first letter.
A string of three English letters is formed as per the following rules:
- The first letter is any vowel.
- The second letter is m, n or p.
- If the second letter is m, then the third letter is any vowel which is different from the first letter.
- If the second letter is n, then the third letter is e or u.
- If the second letter is p, then the third letter is the same as the first letter.
Question 50.
How many strings of letters can possibly be formed using the above rules such that the third letter of the string is e?
Instructions
Answer the question based on the information given below:
Alphonso, on his death bed, keeps half his property for his wife and divide the rest equally among his three sons Ben, Carl and Dave. Some years later Ben dies leaving half his property to his widow and half to his brothers Carl and Dave together, shared equally. When Carl makes his will he keeps half his property for his widow and the rest he bequeaths to his younger brother Dave. When Dave dies some years later, he keeps half his property for his widow and the remaining for his mother. The mother now has Rs. 1,575,000.
Answer the question based on the information given below:
Alphonso, on his death bed, keeps half his property for his wife and divide the rest equally among his three sons Ben, Carl and Dave. Some years later Ben dies leaving half his property to his widow and half to his brothers Carl and Dave together, shared equally. When Carl makes his will he keeps half his property for his widow and the rest he bequeaths to his younger brother Dave. When Dave dies some years later, he keeps half his property for his widow and the remaining for his mother. The mother now has Rs. 1,575,000.
Question 51.
What was the worth of the total property?
Question 52.
A change making machine contains 1 rupee, 2 rupee and 5 rupee coins. The total number of coins is 300. The amount is Rs. 960. If the number of 1 rupee coins and the number of 2 rupee coins are interchanged, the value comes down by Rs. 40. The total number of 5 rupee coins is
Question 53.
In a six-digit number, the sixth, that is, the rightmost, digit is the sum of the first three digits, the fifth digit is the sum of first two digits, the third digit is equal to the first digit, the second digit is twice the first digit and the fourth digit is the sum of fifth and sixth digits. Then, the largest possible value of the fourth digit is
Question 54.
A transport company charges for its vehicles in the following manner:If the driving is 5 hours or less, the company charges Rs. 60 per hour or Rs. 12 per km (which ever is larger) If driving is more than 5 hours, the company charges Rs. 50 per hour or Rs. 7.5 per km (which ever is larger)If Anand drove it for 30 km and paid a total of Rs. 300, then for how many hours does he drive?
Instructions
Directions: Answer the questions based on the following information.
Recently, Ghosh Babu spent his winter vacation on Kyakya Island. During the vacation, he visited the local casino where he came across a new card game. Two players, using a normal deck of 52 playing cards, play this game. One player is called the ‘dealer’ and the other is called the ‘player’. First, the player picks a card at random from the deck. This is called the base card. The amount in rupees equal to the face value of the base card is called the base amount. The face values of ace, king, queen and jack are ten. For other cards the face value is the number on the card. Once the ‘player’ picks a card from the deck, the ‘dealer’ pays him the base amount. Then the ‘dealer’ picks a card from the deck and this card is called the top card. If the top card is of the same suit as the base card, the ‘player’ pays twice the base amount to the ‘dealer’. If the top card is of the same colour as the base card (but not the same suit), then the ‘player’ pays the base amount to the ‘dealer’. If the top card happens to be of a different colour than the base card, the ‘dealer’ pays the base amount to the ‘player’.
Ghosh Babu played the game four times. First time he picked eight of clubs and the ‘dealer’ picked queen of clubs. Second time, he picked ten of hearts and the ‘dealer’ picked two of spades. Next time, Ghosh Babu picked six of diamonds and the ‘dealer’ picked ace of hearts. Lastly, he picked eight of spades and the ‘dealer’ picked jack of spades. Answer the following questions based on these four games.
Directions: Answer the questions based on the following information.
Recently, Ghosh Babu spent his winter vacation on Kyakya Island. During the vacation, he visited the local casino where he came across a new card game. Two players, using a normal deck of 52 playing cards, play this game. One player is called the ‘dealer’ and the other is called the ‘player’. First, the player picks a card at random from the deck. This is called the base card. The amount in rupees equal to the face value of the base card is called the base amount. The face values of ace, king, queen and jack are ten. For other cards the face value is the number on the card. Once the ‘player’ picks a card from the deck, the ‘dealer’ pays him the base amount. Then the ‘dealer’ picks a card from the deck and this card is called the top card. If the top card is of the same suit as the base card, the ‘player’ pays twice the base amount to the ‘dealer’. If the top card is of the same colour as the base card (but not the same suit), then the ‘player’ pays the base amount to the ‘dealer’. If the top card happens to be of a different colour than the base card, the ‘dealer’ pays the base amount to the ‘player’.
Ghosh Babu played the game four times. First time he picked eight of clubs and the ‘dealer’ picked queen of clubs. Second time, he picked ten of hearts and the ‘dealer’ picked two of spades. Next time, Ghosh Babu picked six of diamonds and the ‘dealer’ picked ace of hearts. Lastly, he picked eight of spades and the ‘dealer’ picked jack of spades. Answer the following questions based on these four games.
Question 55.
The initial money Ghosh Babu had (before the beginning of the game sessions) was Rs. X. At no point did he have to borrow any money. What is the minimum possible value of X?
Instructions
Answer the question based on the information given below:
Alphonso, on his death bed, keeps half his property for his wife and divide the rest equally among his three sons Ben, Carl and Dave. Some years later Ben dies leaving half his property to his widow and half to his brothers Carl and Dave together, shared equally. When Carl makes his will he keeps half his property for his widow and the rest he bequeaths to his younger brother Dave. When Dave dies some years later, he keeps half his property for his widow and the remaining for his mother. The mother now has Rs. 1,575,000.
Answer the question based on the information given below:
Alphonso, on his death bed, keeps half his property for his wife and divide the rest equally among his three sons Ben, Carl and Dave. Some years later Ben dies leaving half his property to his widow and half to his brothers Carl and Dave together, shared equally. When Carl makes his will he keeps half his property for his widow and the rest he bequeaths to his younger brother Dave. When Dave dies some years later, he keeps half his property for his widow and the remaining for his mother. The mother now has Rs. 1,575,000.
Question 56.
What was Carl’s original share?
Question 57.
shop stores x kg of rice. The first customer buys half this amount plus half a kg of rice. The second customer buys half the remaining amount plus half a kg of rice. Then the third customer also buys half the remaining amount plus half a kg of rice. Thereafter, no rice is left in the shop. Which of the following best describes the value of x?
Instructions
Choose 1; if the question can be answered by using one of the statements alone, but cannot be answered using the other statement alone.
Choose 2; if the question can be answered by using either statement alone.
Choose 3; if the question can be answered by using both statements together, but cannot be answered using either statement alone.
Choose 4; if the question cannot be answered even by using both statements together.
Two friends, Ram and Gopal, bought apples from a wholesale dealer. How many apples did they buy?
- Ram bought one-half the number of apples that Gopal bought.
- The wholesale dealer had a stock of 500 apples.
Choose 1; if the question can be answered by using one of the statements alone, but cannot be answered using the other statement alone.
Choose 2; if the question can be answered by using either statement alone.
Choose 3; if the question can be answered by using both statements together, but cannot be answered using either statement alone.
Choose 4; if the question cannot be answered even by using both statements together.
Two friends, Ram and Gopal, bought apples from a wholesale dealer. How many apples did they buy?
- Ram bought one-half the number of apples that Gopal bought.
- The wholesale dealer had a stock of 500 apples.
Question 58.
Instructions
Directions: Answer the questions based on the following information.
Recently, Ghosh Babu spent his winter vacation on Kyakya Island. During the vacation, he visited the local casino where he came across a new card game. Two players, using a normal deck of 52 playing cards, play this game. One player is called the ‘dealer’ and the other is called the ‘player’. First, the player picks a card at random from the deck. This is called the base card. The amount in rupees equal to the face value of the base card is called the base amount. The face values of ace, king, queen and jack are ten. For other cards the face value is the number on the card. Once the ‘player’ picks a card from the deck, the ‘dealer’ pays him the base amount. Then the ‘dealer’ picks a card from the deck and this card is called the top card. If the top card is of the same suit as the base card, the ‘player’ pays twice the base amount to the ‘dealer’. If the top card is of the same colour as the base card (but not the same suit), then the ‘player’ pays the base amount to the ‘dealer’. If the top card happens to be of a different colour than the base card, the ‘dealer’ pays the base amount to the ‘player’.
Ghosh Babu played the game four times. First time he picked eight of clubs and the ‘dealer’ picked queen of clubs. Second time, he picked ten of hearts and the ‘dealer’ picked two of spades. Next time, Ghosh Babu picked six of diamonds and the ‘dealer’ picked ace of hearts. Lastly, he picked eight of spades and the ‘dealer’ picked jack of spades. Answer the following questions based on these four games.
Directions: Answer the questions based on the following information.
Recently, Ghosh Babu spent his winter vacation on Kyakya Island. During the vacation, he visited the local casino where he came across a new card game. Two players, using a normal deck of 52 playing cards, play this game. One player is called the ‘dealer’ and the other is called the ‘player’. First, the player picks a card at random from the deck. This is called the base card. The amount in rupees equal to the face value of the base card is called the base amount. The face values of ace, king, queen and jack are ten. For other cards the face value is the number on the card. Once the ‘player’ picks a card from the deck, the ‘dealer’ pays him the base amount. Then the ‘dealer’ picks a card from the deck and this card is called the top card. If the top card is of the same suit as the base card, the ‘player’ pays twice the base amount to the ‘dealer’. If the top card is of the same colour as the base card (but not the same suit), then the ‘player’ pays the base amount to the ‘dealer’. If the top card happens to be of a different colour than the base card, the ‘dealer’ pays the base amount to the ‘player’.
Ghosh Babu played the game four times. First time he picked eight of clubs and the ‘dealer’ picked queen of clubs. Second time, he picked ten of hearts and the ‘dealer’ picked two of spades. Next time, Ghosh Babu picked six of diamonds and the ‘dealer’ picked ace of hearts. Lastly, he picked eight of spades and the ‘dealer’ picked jack of spades. Answer the following questions based on these four games.
Question 59.
If the final amount of money that Ghosh Babu had with him was Rs. 100, what was the initial amount he had with him?
Question 60.
A string of length 40 metres is divided into three parts of different lengths. The first part is three times the second part, and the last part is 23 metres smaller than the first part. Find the length of the largest part.
Question 61.
A yearly payment to the servant is Rs. 90 plus one turban. The servant leaves the job after 9 months and receives Rs. 65 and a turban. Then find the price of the turban.
Instructions
A, B, C are three numbers. Let
@ (A, B) = average of A and B,
/ (A, B) = product of A and B, and
X (A, B) = the result of dividing A by
A, B, C are three numbers. Let
@ (A, B) = average of A and B,
/ (A, B) = product of A and B, and
X (A, B) = the result of dividing A by
Question 62.
The sum of A and B is given by
Question 63.
Mayank, Mirza, Little and Jagbir bought a motorbike for $60. Mayank contributed half of the total amount contributed by others, Mirza contributed one-third of total amount contributed by others, and Little contributed one-fourth of the total amount contributed by others. What was the money paid by Jagbir?
Instructions
Answer the question based on the information given below:
Alphonso, on his death bed, keeps half his property for his wife and divide the rest equally among his three sons Ben, Carl and Dave. Some years later Ben dies leaving half his property to his widow and half to his brothers Carl and Dave together, shared equally. When Carl makes his will he keeps half his property for his widow and the rest he bequeaths to his younger brother Dave. When Dave dies some years later, he keeps half his property for his widow and the remaining for his mother. The mother now has Rs. 1,575,000.
Answer the question based on the information given below:
Alphonso, on his death bed, keeps half his property for his wife and divide the rest equally among his three sons Ben, Carl and Dave. Some years later Ben dies leaving half his property to his widow and half to his brothers Carl and Dave together, shared equally. When Carl makes his will he keeps half his property for his widow and the rest he bequeaths to his younger brother Dave. When Dave dies some years later, he keeps half his property for his widow and the remaining for his mother. The mother now has Rs. 1,575,000.
Question 64.
What was the ratio of the property owned by the widows of the three sons, in the end?
Question 65.
Suppose you have a currency, named Miso, in three denominations: 1 Miso, 10 Misos and 50 Misos. In how many ways can you pay a bill of 107 Misos?
Question 66.
A man received a cheque. The amount in Rs. has been transposed for paise and vice versa. After spending Rs. 5 and 42 paise, he discovered he now had exactly 6 times the value of the correct cheque amount. What amount he should have received?
Question 67.
My son adores chocolates. He likes biscuits. But he hates apples. I told him that he can buy as many chocolates he wishes. But then he must have biscuits twice the number of chocolates and should have apples more than biscuits and chocolates together. Each chocolate cost Re 1. The cost of apple is twice the chocolate and four biscuits are worth one apple. Then which of the following can be the amount that I spent on that evening on my son if number of chocolates, biscuits and apples brought were all integers?
Question 68.
A confused bank teller transposed the rupees and paise when he cashed a cheque for Shailaja, giving her rupees instead of paise and paise instead of rupees. After buying a toffee for 50 paise, Shailaja noticed that she was left with exactly three times as much as the amount on the cheque. Which of the following is a valid statement about the cheque amount?
Instructions
A, B, C are three numbers. Let
@ (A, B) = average of A and B,
/ (A, B) = product of A and B, and
X (A, B) = the result of dividing A by
A, B, C are three numbers. Let
@ (A, B) = average of A and B,
/ (A, B) = product of A and B, and
X (A, B) = the result of dividing A by

