| Test Name | No. of Questions | Marks (of each) | Time | Take Test |
|---|---|---|---|---|
| Test-1 | 10 | 3 | 40 Minutes | |
| Test-2 | 10 | 3 | 40 Minutes | |
| Test-3 | 10 | 3 | 40 Minutes | |
| Test-4 | 10 | 3 | 40 Minutes | |
| Test-5 | 10 | 3 | 40 Minutes | |
| Test-6 | 10 | 3 | 40 Minutes | |
| Test-7 | 10 | 3 | 40 Minutes | |
| Test-8 | 10 | 3 | 40 Minutes |
Question 1.
Suppose f(x, y) is a real-valued function such that f(3x + 2y, 2x - 5y) = 19x, for all real numbers x and y. The value of x for which f(x, 2x) = 27, is
Question 2.
For any real number x, let [x] be the largest integer less than or equal to x. If [1/5 +n/25]= 25, then N is
Question 3.
Suppose for all integers x, there are two functions f and g such that f(x) + f(x - 1) - 1 = 0 and g(x) = x2. If f(x2 - x) = 5, then the value of the sum f(g(5)) + g(f(5)) is
Instructions
Let r be a real number and f(x) = { 2x-r if x r
{ r if x<r
Then, the equation f(x) = f(f(x)) holds for all real values of x where
Let r be a real number and f(x) = { 2x-r if x r
{ r if x<r
Then, the equation f(x) = f(f(x)) holds for all real values of x where
Question 4.
Question 5.
f(x) = is negative if and only if
Question 6.
If f(x) = x2 – 7x and g(x) = x + 3, then the minimum value of f(g(x)) – 3x is
Question 7.
Among 100 students, have birthdays in January, have birthdays in February, and so on. If = max(x1, x2, …., x12), then the smallest possible value of is
Question 8.
If f(5 + x) = f(5 - x) for every real x, and f(x) = 0 has four distinct real roots, then the sum of these roots is
Question 9.
The area of the region satisfying the inequalities |x| - y ≤ 1, y ≥ 0 and y ≤ 1 is
Question 10.
Let f(x) = x² + ax + b and g(x) = f(x + 1) – f(x – 1). If f(x) ≥ 0 for all real x, and g(20) = 72, then the smallest possible value of b is
Question 11.
If f(x + y) = f(x)f(y) and f(5) = 4, then f(10) – f(-10) is equal to
Question 12.
For any positive integer n, let f(n) = n(n + 1) if n is even, and f(n) = n + 3 if n is odd. If m is a positive integer such that 8 f(m + 1) − f(m) = 2, then m equals
Question 13.
Consider a function f satisfying f(x + y) = f(x) f(y) where x, y are positive integers and f(1) = 2. If f(a + 1) + f(a + 2) +…+ f(a + n) = 16(2n – 1) then a is equal to
Question 14.
Let f be a function such that f(mn) = f(m) × f(n) for every positive integers m and n. If f(1), f(2) and f(3) are positive integers, f(1) < f(2), and f(24) = 54, then f(18) equals
Question 15.
If f(x + 2) = f(x) + f(x + 1) for all positive integers x, and f(11) = 91, f(15) = 617, then f(10) equals
Question 16.
Let f(x) = min {2x2, 52 − 5x}, where x is any positive real number. Then the maximum possible value of f(x) is
Question 17.
let f(x) = max {5x, 52 – 2x2}, where x is any positive real number. Then the minimum possible value of f(x) is
Question 18.
The shortest distance of the point (1/2,1) from the curve y = |x - 1| + |x + 1| is
Question 19.
f f(x) = (5x+2)/(3x-5) and g(x) = x2 – 2x – 1, then the value of g(f(f(3))) is:
Question 20.
Let f(x) = x2 and g(x) = 2x, for all real x. Then the value of f(f(g(x)) + g(f(x))) at x = 1 is
Question 21.
If f(ab) = f(a)f(b) for all positive integers a and b, then the largest possible value of f(1) is
Question 22.
Let f(x) = 2x – 5 and g(x) = 7 – 2x. Then |f(x) + g(x)| = |f(x)| + |g(x)| if and only if
Question 23.
What is the value of a + b + c?
Question 24.
Let f(x) be a function satisfying f(x) × f(y) = f(xy) for all real x, y. Let f(2) = 4, then what is the value of f(1/2) ?
Question 25.
A function f(x) satisfies f(1) = 3600, and f(1) + f(2) + ... + f(n) = n²f(n), for all positive integers n > 1. What is the value of f(9)?
Instructions
The graph of y – x against y + x is as shown below. (All graphs in this question are drawn to scale and the same scale has been used on each axis). Then, which of the options given shows the graph of y against x.
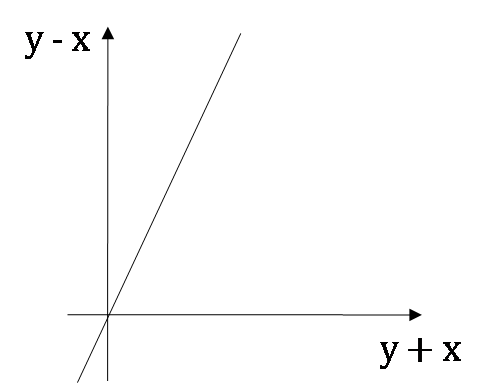
a) 
b) 
c) 
d) 
The graph of y – x against y + x is as shown below. (All graphs in this question are drawn to scale and the same scale has been used on each axis). Then, which of the options given shows the graph of y against x.
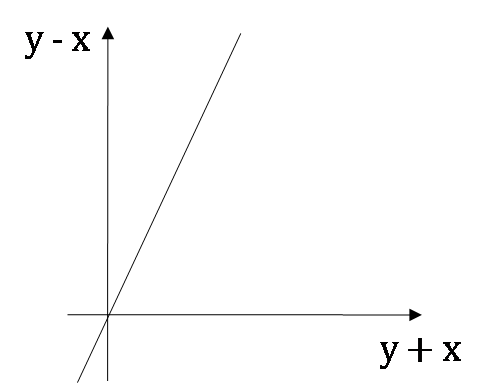
a) 
b) 
c) 
d) 
Question 26.
Question 27.
Let f(x) = max (2x + 1, 3 − 4x), where x is any real number. Then the minimum possible value of f(x) is:
Question 28.
In the X-Y plane, the area of the region bounded by the graph of |x + y| + |x – y| = 4 is
Question 29.
Let g(x) be a function such that g(x + 1) + g(x – 1) = g(x) for every real x. Then for what value of p is the relation g(x + p) = g(x) necessarily true for every real x?
Question 30.
Let f(x) = ax^2– b|x|, where a and b are constants. Then at x = 0, f(x) is
Question 31.
How many of the following products are necessarily zero for every xf1(x)f2(x), f2(x)f3(x), f2(x)f4(x)?
Question 32.
Which of the following is necessarily true?
Question 33.
The number of non-negative real roots of 2x – x – 1 = 0 equals
Question 34.
When the curves, y = log10 x and y = x−1 are drawn in the X-Y plane, how many times do they intersect for values of x ≥ 1?
Question 35.
Let g(x) = max(5 − x, x + 2). The smallest possible value of g(x) is
Question 36.
The function f(x) = |x − 2| + |2.5 − x| + |3.6 − x|, where x is a real number, attains a minimum at
Question 37.
Consider the following two curves in the X-Y planey = x3 + x2 + 5y = x2 + x + 5Which of the following statements is true for −2 ≤ x ≤ 2?
Question 38.
If f ( x ) = \log(\1+x/1-x) , then f(x) + f(y) =
Question 39.
Suppose, for any real number x, [x] denotes the greatest integer less than or equal to x. Let L(x, y) = [x] + [y] + [x + y] and R(x, y) = [2x] + [2y]. Then it’s impossible to find any two positive real numbers x and y for which of the following?
Instructions

In the above table, for suitably chosen constants a, b and c, which one of the following best describes the relation between y and x?

In the above table, for suitably chosen constants a, b and c, which one of the following best describes the relation between y and x?
Question 40.
Question 41.
Which of the following expressions yields a positive value for every pair of non-zero real number (x, y)?
Question 42.
Under which of the following conditions is f(x, y) necessarily greater than g(x, y)?
Question 43.
Which of the following expressions is necessarily equal to 1?
Question 44.
Which of the following expressions is indeterminate?
Instructions
Given below are three graphs made up of straight-line segments shown as thick lines. In each case choose the answer as
1. if f(x) = 3 f(–x);
2. if f(x) = –f(–x);
3. if f(x) = f(–x); and
4. if 3 f(x) = 6 f(–x), for x ≥ 0.

Given below are three graphs made up of straight-line segments shown as thick lines. In each case choose the answer as
1. if f(x) = 3 f(–x);
2. if f(x) = –f(–x);
3. if f(x) = f(–x); and
4. if 3 f(x) = 6 f(–x), for x ≥ 0.

Question 45.
Instructions
Given below are three graphs made up of straight-line segments shown as thick lines. In each case choose the answer as
1. if f(x) = 3 f(–x);
2. if f(x) = –f(–x);
3. if f(x) = f(–x); and
4. if 3 f(x) = 6 f(–x), for x ≥ 0.
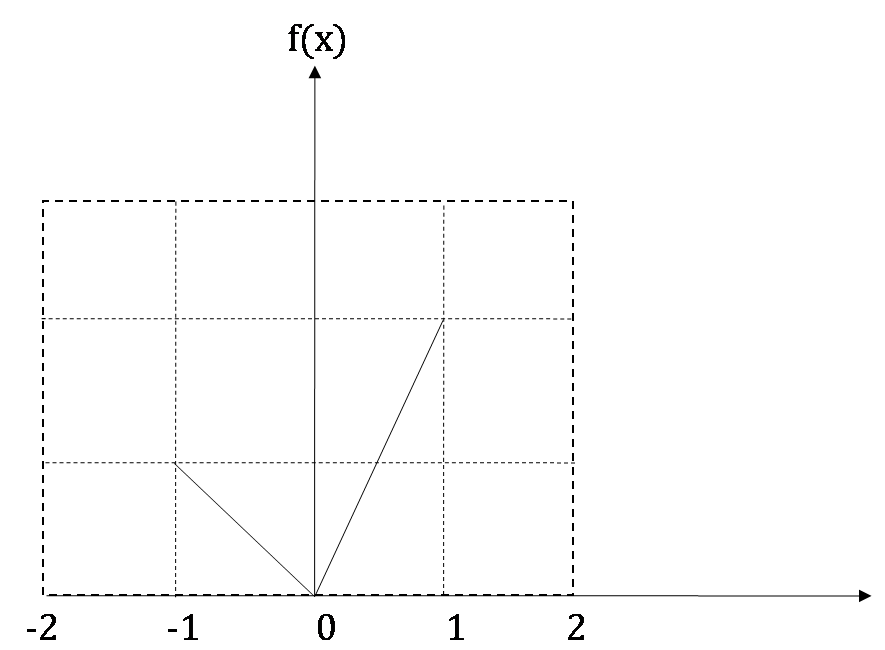
Given below are three graphs made up of straight-line segments shown as thick lines. In each case choose the answer as
1. if f(x) = 3 f(–x);
2. if f(x) = –f(–x);
3. if f(x) = f(–x); and
4. if 3 f(x) = 6 f(–x), for x ≥ 0.
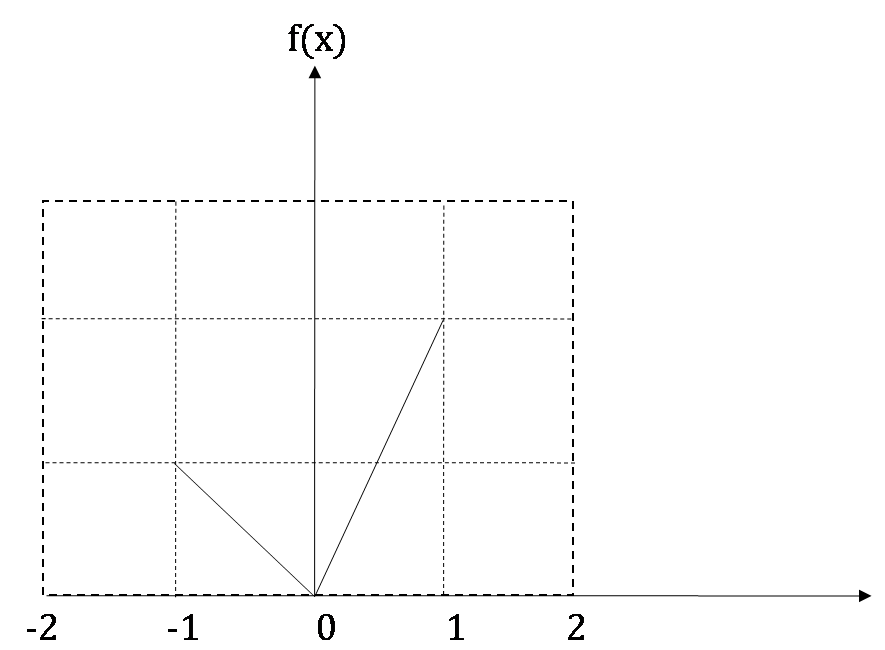
Question 46.
Instructions
Given below are three graphs made up of straight-line segments shown as thick lines. In each case choose the answer as
1. if f(x) = 3 f(–x);
2. if f(x) = –f(–x);
3. if f(x) = f(–x); and
4. if 3 f(x) = 6 f(–x), for x ≥ 0.
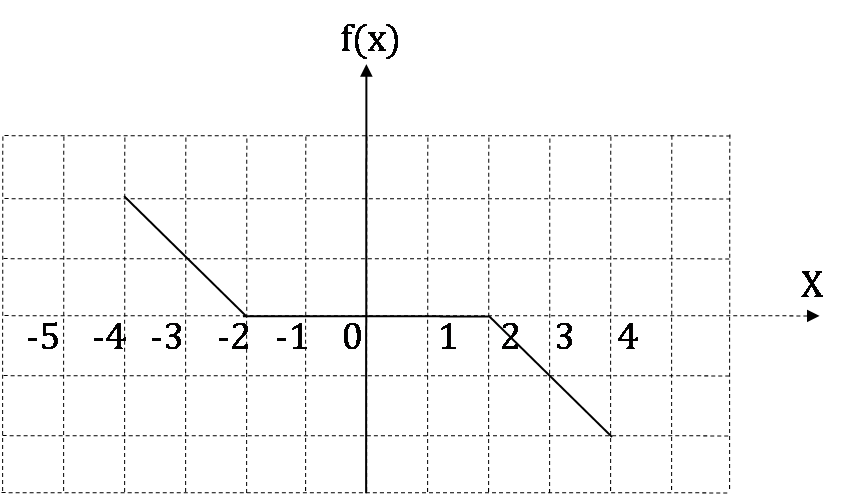
Given below are three graphs made up of straight-line segments shown as thick lines. In each case choose the answer as
1. if f(x) = 3 f(–x);
2. if f(x) = –f(–x);
3. if f(x) = f(–x); and
4. if 3 f(x) = 6 f(–x), for x ≥ 0.
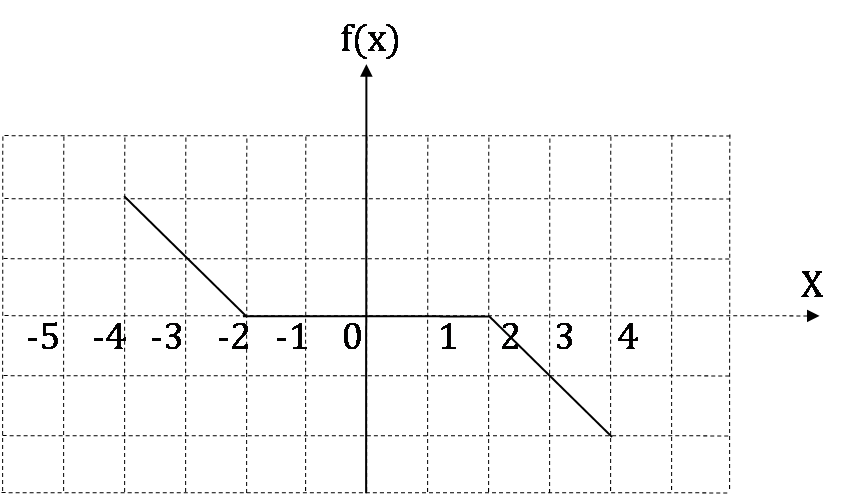
Question 47.
Instructions
For a real number x, let
f(x) = 1/(1 + x), if x is non-negative
= 1+ x, if x is negative
f n(x) = f(f n – 1(x)), n = 2, 3, ....
What is the value of the product, f(2)f2(2)f3(2)f4(2)f5(2)?
For a real number x, let
f(x) = 1/(1 + x), if x is non-negative
= 1+ x, if x is negative
f n(x) = f(f n – 1(x)), n = 2, 3, ....
What is the value of the product, f(2)f2(2)f3(2)f4(2)f5(2)?
Question 48.
Instructions
r is an integer > 2. Then, what is the value of f r – 1(–r) + f r(–r)+ f r + 1 (–r)?
r is an integer > 2. Then, what is the value of f r – 1(–r) + f r(–r)+ f r + 1 (–r)?
Question 49.
Instructions
The set of all positive integers is the union of two disjoint subsets
{f(1), f(2) ....f(n),......} and {g(1), g(2),......,g(n),......}, where
f (1) < f(2) <...< f(n) ....., and g(1) < g(2) <...< g(n) ......., and
g(n) = f(f(n)) + 1 for all n ≥ 1.
What is the value of g(1)?
The set of all positive integers is the union of two disjoint subsets
{f(1), f(2) ....f(n),......} and {g(1), g(2),......,g(n),......}, where
f (1) < f(2) <...< f(n) ....., and g(1) < g(2) <...< g(n) ......., and
g(n) = f(f(n)) + 1 for all n ≥ 1.
What is the value of g(1)?
Question 50.
Question 51.
For all non-negative integers x and y, f(x, y) is defined as belowf(0, y) = y + 1f(x + 1, 0) = f(x, 1)f(x + 1,y + 1) = f(x, f(x + 1, y))Then, what is the value of f(1, 2)?
Instructions
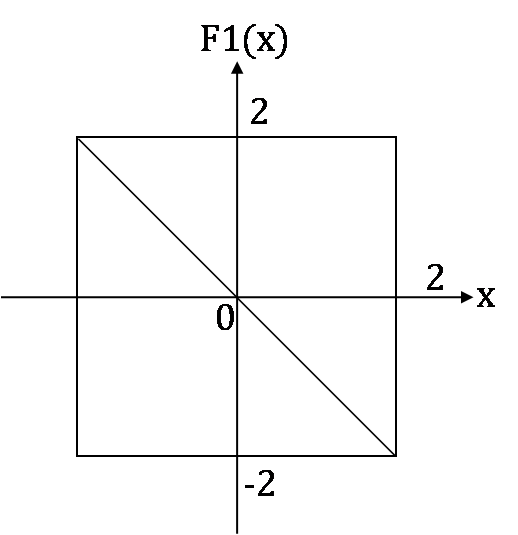

In each of the following questions, a pair of graphs F(x) and F1(x) is given. These are composed of straightline segments, shown as solid lines, in the domain x ∈ (−2, 2).
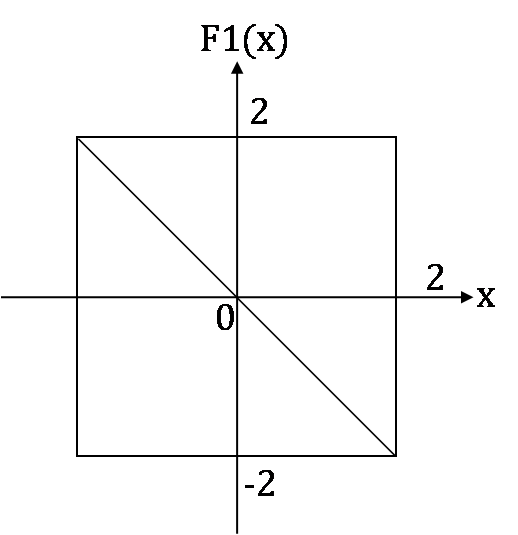

In each of the following questions, a pair of graphs F(x) and F1(x) is given. These are composed of straightline segments, shown as solid lines, in the domain x ∈ (−2, 2).
Question 52.
Instructions
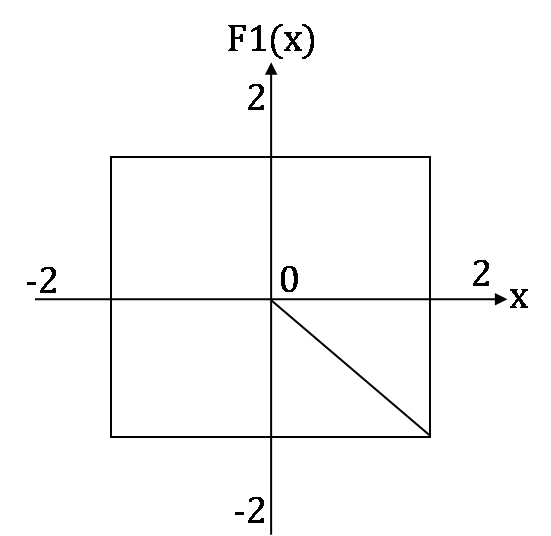

In each of the following questions, a pair of graphs F(x) and F1(x) is given. These are composed of straightline segments, shown as solid lines, in the domain x ∈ (−2, 2).


In each of the following questions, a pair of graphs F(x) and F1(x) is given. These are composed of straightline segments, shown as solid lines, in the domain x ∈ (−2, 2).
Question 53.
Instructions


In each of the following questions, a pair of graphs F(x) and F1(x) is given. These are composed of straightline segments, shown as solid lines, in the domain x ∈ (−2, 2).


In each of the following questions, a pair of graphs F(x) and F1(x) is given. These are composed of straightline segments, shown as solid lines, in the domain x ∈ (−2, 2).
Question 54.
Instructions
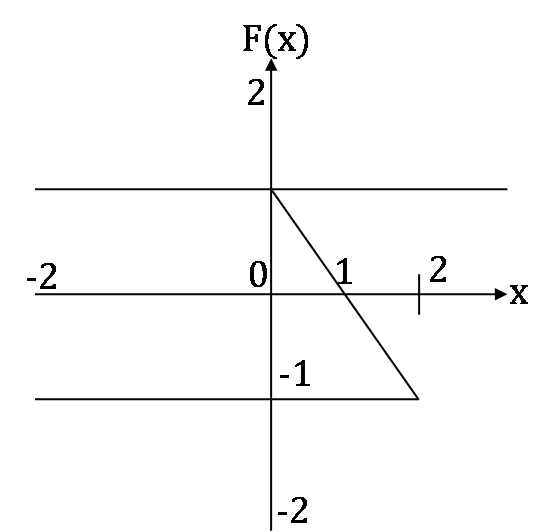
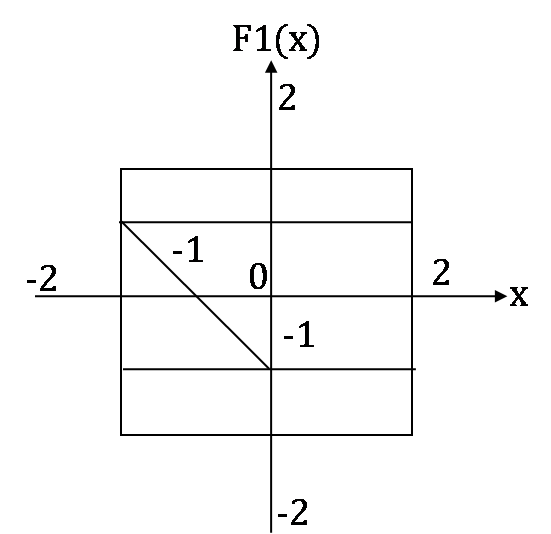
In each of the following questions, a pair of graphs F(x) and F1(x) is given. These are composed of straightline segments, shown as solid lines, in the domain x ∈ (−2, 2).
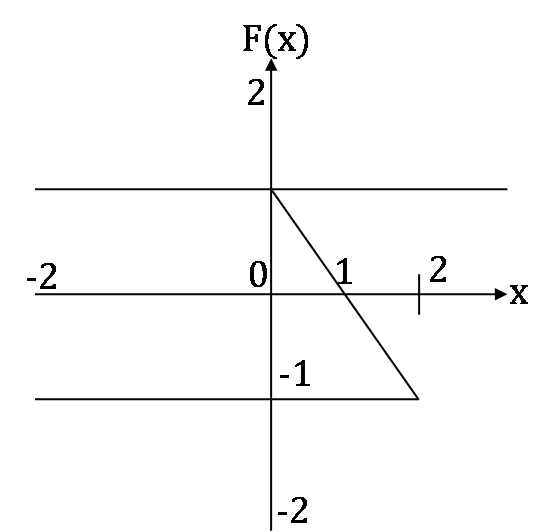

In each of the following questions, a pair of graphs F(x) and F1(x) is given. These are composed of straightline segments, shown as solid lines, in the domain x ∈ (−2, 2).
Question 55.
Question 56.
Which of the following statements is true?
Question 57.
What is the value of f(G(f(1, 0)), f(F(f(1, 2)), G(f(1, 2))))?
Question 58.
Which of the following expressions yields x2 as its result?
Instructions
la(x, y, z) = min(x + y, y + z)
le(x, y, z) = max (x − y, y − z)
ma(x, y, z) = 1/2[le(x, y, z) + la(x, y, z)]
Given that x > y > z > 0. Which of the following is necessarily true?
la(x, y, z) = min(x + y, y + z)
le(x, y, z) = max (x − y, y − z)
ma(x, y, z) = 1/2[le(x, y, z) + la(x, y, z)]
Given that x > y > z > 0. Which of the following is necessarily true?
Question 59.
Instructions
la(x, y, z) = min(x + y, y + z)
le(x, y, z) = max (x − y, y − z)
ma(x, y, z) = 1/2 [le(x, y, z) + la(x, y, z)]
What is the value of ma(10, 4, le(la(10, 5, 3), 5, 3))?
la(x, y, z) = min(x + y, y + z)
le(x, y, z) = max (x − y, y − z)
ma(x, y, z) = 1/2 [le(x, y, z) + la(x, y, z)]
What is the value of ma(10, 4, le(la(10, 5, 3), 5, 3))?
Question 60.
Question 61.
For x = 15, y = 10 and z = 9 , find the value of le(x, min(y, x − z), le (9, 8, ma(x, y, z))).
Instructions
A, S, M and D are functions of x and y, and they are defined as follows.
A(x, y) = x + y
S(x, y) = x – y
M(x, y) = xy
D(x, y) = x/y , y ≠ 0
A, S, M and D are functions of x and y, and they are defined as follows.
A(x, y) = x + y
S(x, y) = x – y
M(x, y) = xy
D(x, y) = x/y , y ≠ 0
Question 62.
What is the value of M(M(A(M(x, y), S(y, x)), x), A(y, x)) for x = 2, y = 3?
Instructions
A, S, M and D are functions of x and y, and they are defined as follows.
A(x, y) = x + y
S(x, y) = x – y
M(x, y) = xy
D(x, y) = x/y , y ≠ 0
A, S, M and D are functions of x and y, and they are defined as follows.
A(x, y) = x + y
S(x, y) = x – y
M(x, y) = xy
D(x, y) = x/y , y ≠ 0
Question 63.
What is the value of S[M(D(A(a, b), 2), D(A(a, b), 2)), M(D(S(a, b), 2), D(S(a, b), 2))]?
Instructions
Largest value of min(2 + x2, 6 – 3x), when x > 0, is
Largest value of min(2 + x2, 6 – 3x), when x > 0, is
Question 64.
Instructions
le(x, y) = Least of (x, y)
mo(x) = |x|
me(x, y) = Maximum of (x, y)
le(x, y) = Least of (x, y)
mo(x) = |x|
me(x, y) = Maximum of (x, y)
Question 65.
Find the value of me(a + mo(le(a, b)); mo(a + me(mo(a), mo(b))), at a = –2 and b = –3.
Instructions
le(x, y) = Least of (x, y)
mo(x) = |x|
me(x, y) = Maximum of (x, y)
le(x, y) = Least of (x, y)
mo(x) = |x|
me(x, y) = Maximum of (x, y)
Question 66.
Which of the following must always be correct for a, b > 0?
Instructions
le(x, y) = Least of (x, y)
mo(x) = |x|
me(x, y) = Maximum of (x, y)
For what values of ‘a’ is me(a2 – 3a, a – 3) < 0?
le(x, y) = Least of (x, y)
mo(x) = |x|
me(x, y) = Maximum of (x, y)
For what values of ‘a’ is me(a2 – 3a, a – 3) < 0?
Question 67.
Instructions
le(x, y) = Least of (x, y)
mo(x) = |x|
me(x, y) = Maximum of (x, y)
For what values of ‘a’ is le(a2 – 3a, a – 3) < 0?
le(x, y) = Least of (x, y)
mo(x) = |x|
me(x, y) = Maximum of (x, y)
For what values of ‘a’ is le(a2 – 3a, a – 3) < 0?
Question 68.
Instructions
If
md(x) = x ,
mn(x,y) = minimum of x and y and
Ma(a,b,c,...) = maximum of a,b,c…
If
md(x) = x ,
mn(x,y) = minimum of x and y and
Ma(a,b,c,...) = maximum of a,b,c…
Question 69.
Value of Ma[md(a),mn(md(b),a),mn(ab,md(ac))] where a = -2, b = -3, c = 4 is
Instructions
If
md(x) = x ,
mn(x,y) = minimum of x and y and
Ma(a,b,c,...) = maximum of a,b,c…
If
md(x) = x ,
mn(x,y) = minimum of x and y and
Ma(a,b,c,...) = maximum of a,b,c…
Question 70.
Given that a > b then the relation Ma[md(a), mn(a,b)] = mn[a, md(Ma(a,b))] does not hold if
Instructions
If f (x) = 2x + 3 and g(x) = (x-3)/2 , then
If f (x) = 2x + 3 and g(x) = (x-3)/2 , then
Question 71.
fog(x) is equal to
Instructions
If f (x) = 2x + 3 and g(x) = (x-3)/2 , then
If f (x) = 2x + 3 and g(x) = (x-3)/2 , then
Question 72.
For what value of x; f (x) = g(x −3)?
Instructions
If f (x) = 2x + 3 and g(x) = (x-3)/2 , then
If f (x) = 2x + 3 and g(x) = (x-3)/2 , then
Question 73.
What is the value of (gofofogogof)(x) × (fogofog)(x)?
Instructions
If f (x) = 2x + 3 and g(x) = (x-3)/2 , then
If f (x) = 2x + 3 and g(x) = (x-3)/2 , then
Question 74.
What is the value of fo(fog)o(gof)(x)?
Instructions
A function f(x) is said to be even if f(–x) = f(x), and odd if f(–x) = –f(x). Thus, for example, the function given by f(x) = x2 is even, while the function given by f(x) = x3 is odd. Using this definition, answer the following questions.
The function given by f(x) = |x|3 is
A function f(x) is said to be even if f(–x) = f(x), and odd if f(–x) = –f(x). Thus, for example, the function given by f(x) = x2 is even, while the function given by f(x) = x3 is odd. Using this definition, answer the following questions.
The function given by f(x) = |x|3 is
Question 75.
Instructions
A function f(x) is said to be even if f(–x) = f(x), and odd if f(–x) = –f(x). Thus, for example, the function given by f(x) = x2 is even, while the function given by f(x) = x3 is odd. Using this definition, answer the following questions.
The sum of two odd functions
A function f(x) is said to be even if f(–x) = f(x), and odd if f(–x) = –f(x). Thus, for example, the function given by f(x) = x2 is even, while the function given by f(x) = x3 is odd. Using this definition, answer the following questions.
The sum of two odd functions
Question 76.
Instructions
The maximum possible value of y = min (1/2 – 3x2/4, 5x2/4) for the range 0 < x < 1 is
The maximum possible value of y = min (1/2 – 3x2/4, 5x2/4) for the range 0 < x < 1 is
Question 77.
Question 78.
A function can sometimes reflect on itself, i.e. if y = f(x), then x = f(y). Both of them retain the same structure and form. Which of the following functions has this property?
Instructions
If y = f(x) and f(x) = (1-x)/(1+x) , which of the following is true?
If y = f(x) and f(x) = (1-x)/(1+x) , which of the following is true?

